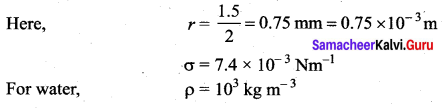Students can Download Physics Chapter 7 Properties of Matter Questions and Answers, Notes Pdf, Samacheer Kalvi 11th Physics Solutions Guide Pdf helps you to revise the complete Tamilnadu State Board New Syllabus and score more marks in your examinations.
Tamilnadu Samacheer Kalvi 11th Physics Solutions Chapter 7 Properties of Matter
Samacheer Kalvi 11th Physics Properties of Matter Textual Evaluation Solved
Samacheer Kalvi 11th Physics Properties of Matter Multiple Choice Questions
Question 1.
Consider two wires X and Y. The radius of wire X is 3 times the radius of Y. If they are stretched by the same load then the stress on Y is ……….
(a) equal to that on X
(b) thrice that on X
(c) nine times that on X
(d) Half that on X
Answer:
(c) nine times that on X
Solution:
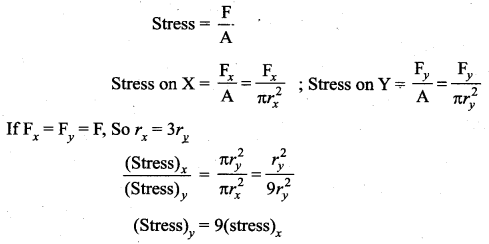
Question 2.
If a wire is streched to double of its original length, then the strain in the wire is ………
(a) 1
(b) 2
(c) 3
(d) 4
Answer:
(d) 4
Solution:

In this case Young’s modulus is equal to stress
![]()
Question 3.
The load-elongation graph of three wires of the same material are shown in figure. Which of the following wire is the thickest?
(a) wire 1
(b) wire 2
(c) wire 3
(d) all of them have same thickness
Answer:
(a) wire 1
Solution:
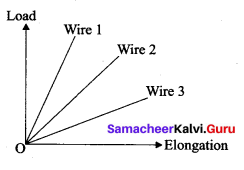
Wire 1 is the thickest compared to other wires.
Because the elongation of the wire 1 is minimum.
![]()
Question 4.
For a given material, the rigidity modulus is \(\left(\frac{1}{3}\right)^{r d}\) of Young’s modulus. Its Poisson’s ratio is
(a) 0
(b) 0.25
(c) 0.3
(d) 0.5
Answer:
(d) 0.5
Solution:
The relationship of Poisson’s ratio, rigidity and Young’s modulus is
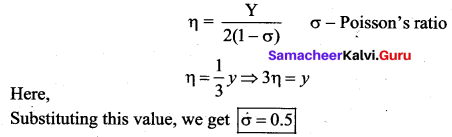
Question 5.
A small sphere of radius 2 cm falls from rest in a viscous liquid. Heat is produced due to viscous force. The rate of production of heat when the sphere attains its terminal velocity is proportional to ……… [NEET model 2018]
(a) 22
(b) 23
(c) 2 4
(d) 25
Answer:
(d) 25
Solution:
Rate of heat produced
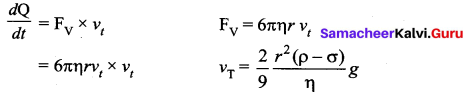
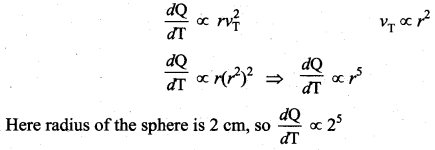
Question 6.
Two wires are made of the same material and have the same volume. The area of cross sections
of the first and the second wires are A and 2A respectively. If the length of the first wire is increased by ∆l on applying a force F, how much force is needed to stretch the second wire by the same amount? (NEET model 2018)
(a) 2
(b) 4
(c) 8
(d) 16
Answer:
(b) 4
Solution:
Since the two wires have same volume
Length of wire 1 = l, Area of wire 1 = A
Length of wire 2 = \(\frac{l}{2}\), Area of wire 2 = 2A

Question 7.
With an increase in temperature, the viscosity of liquid and gas, respectively will
(a) increase and increase
(b) increase and decrease
(c) decrease and increase
(d) decrease and decrease
Answer:
(c) decrease and increase
![]()
Question 8.
The Young’s modulus for a perfect rigid body is ……..
(a) 0
(b) 1
(c) 0.5
(d) infinity
Answer:
(d) infinity
Question 9.
Which of the following is not a scalar?
(a) viscosity
(b) surface tension
(c) pressure
(d) stress
Answer:
(d) stress
Question 10.
If the temperature of the wire is increased, then the Young’s modulus will …….
(a) remain the same
(b) decrease
(c) increase rapidly
(d) increase by very a small amount
Answer:
(b) decrease
Solution:
As temperature is increased, the strain i.e., change dimensions of the body is increased, as a result, the stiffness of the material is reduced. Which causes decrease in magnitude of modulus of elasticity.
Question 11.
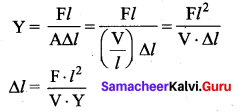
Copper of fixed volume V is drawn into a wire of length l. When this wire is subjected to a constant force F, the extension produced in the wire is ∆l. If Y represents the Young’s modulus, then which of the following graphs is a straight line? [NEET 2014 model]
(a) ∆l versus V
(b) ∆l versus Y
(c) ∆l versus F
(d) ∆l versus \(\frac{1}{l}\)
Answer:
(c) ∆l versus F
Solution:
From Young’s modulus,
Question 12.
A certain number of spherical drops of a liquid of radius R coalesce to form a single drop of radius R and volume V. If T is the surface tension of the liquid, then
Answer:
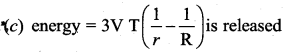
![]()
Question 13.
The following four wires are made of the same material. Which of these will have the largest extension when the same tension is applied?
(a) length = 200 cm, diameter = 0.5 mm
(b) length = 200 cm, diameter = 1 mm
(c) length = 200 cm, diameter = 2 mm
(d) length = 200 cm, diameter = 3 mm
Answer:
(a) length = 200 cm, diameter = 0.5 mm
Solution:
From Young’s modulus,
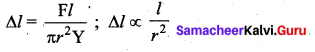
Question 14.
The wettability of a surface by a liquid depends primarily on
(a) viscosity
(b) surface tension
(c) density
(d) angle of contact between the surface and the liquid
Answer:
(d) angle of contact between the surface and the liquid
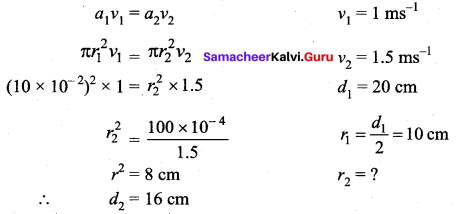
Question 15.
In a horizontal pipe of non-uniform cross section, water flows with a velocity of 1 ms-1 at a point where the diameter of the pipe is 20 cm. The velocity of water 1.5 (ms-1) at a point where the diameter of the pipe is.
(a) 8
(b) 16
(c) 24
(d) 32
Answer:
(b) 16
Solution:
From equation of continuity,
Samacheer Kalvi 11th Physics Properties of Matter Short Answer Questions
Question 1.
Define stress and strain.
Answer:
Stress: The restoring force per unit area of a deformed body is known as stress.
Strain: Strain produced in a body is defined as the ratio of change in size of a body to the original size.
![]()
Question 2.
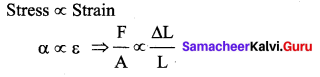
State Hooke’s law of elasticity.
Answer:
The stress is proportional to the strain in the elastic limit.
Question 3.
Define Poisson’s ratio.
Answer:
Poisson’s ratio, which is defined as the ratio of relative contraction (lateral strain) to relative
expansion (longitudinal strain). It is denoted by the symbol μ.
![]()
Question 4.
Explain elasticity using intermolecular forces.
Answer:
Elastic behaviour of solid. In a solid, atoms and molecules are arranged in such a way that each molecule is acted upon by the forces due to the neighbouring molecules. When deforming force is applied on a body so that its length increases, then the molecules of the body go far apart.
Question 5.
Which one of these is more elastic, steel or rubber? Why?
Answer:
Steel is more elastic than rubber. If an equal stress is applied to both steel and rubber, the steel produces less strain. So the Young’s modulus is higher for steel than rubber. The object which has higher young’s modulus is more elastic.
Question 6.
A spring balance shows wrong readings after using for a long time. Why?
Answer:
When a spring balance has been used for a long time, it develops an elastic fatigue, the spring of such a balance take longer time to recover its original configuration and therefore it does not give correct measurement.
![]()
Question 7.
What is the effect of temperature on elasticity?
Answer:
As the temperature of substance increases, its elasticity decreases.
Question 8.
Write down the expression for the elastic potential energy of a stretched wire.
Answer:
The work done in stretching the wire by dl,
dW = F.dl
The total work done in stretching the wire from 0 to l is

From Young’s modulus of elasticity, force becomes,

Substituting equation (2) in (1) we get,

This work done is known as the elastic potential energy of a stretched wire.
Question 9.
State Pascal’s law in fluids.
Answer:
If the pressure in a liquid is changed at a particular point, the change is transmitted to the entire liquid without being diminished in magnitude.
Question 10.
State Archimedes principle.
Answer:
If states that when a body is partially or wholly immersed in a fluid, it experiences an upward thrust equal to the weight of the fluid displaced by it and its upthrust acts through the centre of gravity of the liquid displaced.
Question 11.
What do you mean by upthrust or buoyancy?
Answer:
The upward force exerted by a fluid that opposes the weight of an immersed object in a fluid is called upthrust or buoyant force.
![]()
Question 12.
State the law of floatation.
Answer:
The law of floatation states that a body will float in a liquid if the weight of the liquid displaced by the immersed part of the body equals the weight of the body.
Question 13.
Define coefficient of viscosity of a liquid.
Answer:
The coefficient of viscosity of a liquid is the viscous force acting tangentially per unit area of a liquid layer having a unit velocity gradient in a direction perpendicular to the direction of flow of the liquid.
Question 14.
Distinguish between streamlined flow and turbulent flow.
Answer:
| Streamlined flow | Turbulent Flow |
| When a liquid flow such that each particle of the liquid passing a point moves along the same path and has the same velocity as its predecessor than the flow of liquids is said to be streamlined flow. | During the flow of fluid, when the critical velocity is exceeded by the moving fluid, the motion becomes turbulent. |
Question 15.
What is Reynold’s number? Give its significance.
Answer:
It is a dimensionless number which determines the nature of the flow of fluid through a pipe. Reynold’s number is given by,
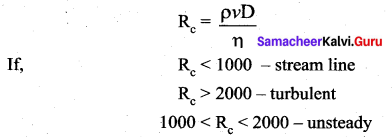
Question 16.
Define terminal velocity.
Answer:
The maximum constant velocity acquired by a body while falling freely through a viscous medium is called the terminal velocity VT.
![]()
Question 17.
Write down the expression for the Stoke’s force and explain the symbols involved in it.
Answer:
The viscous force F acting on a spherical body of radius r depends directly on:
(i) radius (r) of the sphere
(ii) velocity (v) of the sphere and
(iii) coefficient of viscosity η of the liquid
![]()
On solving, we get x = 1, y = 1 and z = 1. Therefore, F = kηrv
Experimentally, Stoke found that the value of k = 6π
F = 6πηrv This relation is known as Stoke’s law.
Question 18.
State Bernoulli’s theorem.
Answer:
It states that the sum of pressure energy, kinetic energy and potential energy per unit volume of an incompressible, non-viscous fluid in a streamlined, irrotational flow remains constant along
a streamline.

Question 19.
What are the energies possessed by a liquid? Write down their equations.
Answer:
A liquid in motion possesses following three types of energy:
(i) Kinetic energy: It is the energy possessed by a liquid by virtue of its motion.
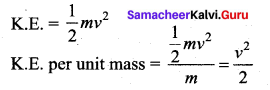
(ii) Potential energy: It is the energy possessed by a liquid by virtue of its height above the ground level.
P.E. = mgh
![]()
(iii) Pressure energy: It is the energy possessed by a liquid by virtue of its pressure. Pressure energy per unit mass = \(\frac{\mathbf{P}}{m / v}\)
Question 20.
Two streamlines cannot cross each other. Why?
Answer:
If two streamlines cross each other, there will be two directions of flow at the point of intersection which is impossible.
![]()
Question 21.
Define surface tension of a liquid. Mention its S.I. Unit and dimension.
Answer:
The surface of a liquid is defined as the, force per unit length of a liquid (or) the energy per unit area of the surface of a liquid. T = \(\frac{\mathbf{F}}{l}\)
SI unit and dimensions of T are Nm-1 and MT-2
Question 22.
How is surface tension related to surface energy?
Answer:
The surface energy per unit area of a surface is numerically equal to the surface tension.
Question 23.
Define angle of contact for a given pair of solid and liquid.
Answer:
The angle between tangents drawn at the point of contact to the liquid surface and solid surface inside the liquid is called the angle of contact for a pair of solid and liquid. It is denoted by θ.
Question 24.
Distinguish between cohesive and adhesive forces.
Answer:
The force between the like molecules which holds the liquid together is called ‘cohesive force’. When the liquid is in contact with a solid, the molecules of the these solid and liquid will experience an attractive force which is called‘adhesive force’.
Question 25.
What are the factors affecting the surface tension of a liquid?
Answer:
- The presence of any contamination or impurities.
- The presence of dissolved substances.
- Electrification
- Temperature
Question 26.
What happens to the pressure inside a soap bubble when air is blown into it?
Answer:
When air is blown into a soap bubble, the pressure inside a bubble is decreased P = \(\frac{4 \mathrm{T}}{\mathrm{R}}\)
![]()
Question 27.
What do you mean by capillarity or capillary action?
Answer:
In a liquid whose angle of contact with solid is less than 90°, suffers capillary rise. On the other hand, in a liquid whose angle of contact is greater than 90°, suffers capillary fall. The rise or fall of a liquid in a narrow tube is called capillarity or capillary action.
Question 28.
A drop of oil placed on the surface of water spreads out. But a drop of water placed on oil contracts to a spherical shape. Why?
Answer:
The surface tension of the water is more than that of oil. Therefore, when oil is poured over water, the greater value of surface tension of water pulls oil in all directions, and as such it spreads on the water. On the other hand, when water is poured over oil, it does not spread over it because the surface tension of oil being less than that of water, it is not able to pull water over it.
Question 29.
State the principle and usage of venturimeter.
Answer:
This device is used to measure the rate of flow (or say flow speed) of the incompressible fluid flowing through a pipe. It works on the principle of Bernoulli’s theorem.
Samacheer Kalvi 11th Physics Properties of Matter Long Answer Questions
Question 1.
State Hooke’s law and verify it with the help of an experiment.
Answer:
Hooke’s law is for a small deformation, when the stress and strain are proportional to each other. It can be verified in a simple way by stretching a thin straight wife (stretches like spring) of length L and uniform cross sectional area A suspended from a fixed point O. A pan and a pointer are attached at the free end of the wire.
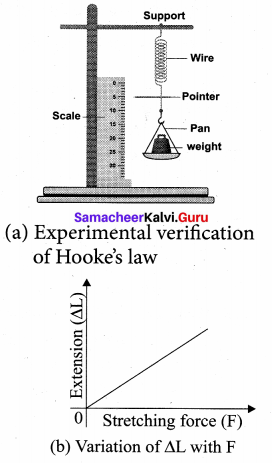
The extension produced on the wire is measured using a vernier scale arrangement. The experiment shows that for a given load, the corresponding stretching force is F and the elongation produced on the wire is ∆L. It is directly proportional to the original length L and inversely proportional to the area of cross section A. A graph is plotted using F on the X-axis and ∆L on the Y-axis.
Therefore ∆L = (slope)F
Multiplying and dividing by volume,
V = AL
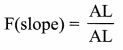

Comparing with stress and strain equations,
σ ∝ ε
i.e., the stress is proportional to the strain in the elastic limit.
Stress-strain profile curve: The stress versus strain profile is a plot in which stress and strain are noted for each load and a graph is drawn taking strain along the X-axis and stress along the Y-axis. The elastic characteristics of the materials can be analyzed from the stress-strain profile.
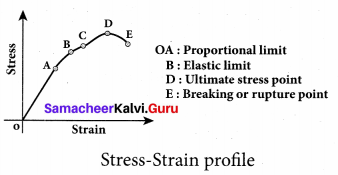
(a) Portion OA: In this region, stress is very small such that stress is proportional to strain, which means Hooke’s law is valid. The point A is called limit of proportionality because above this point Hooke’s law is not valid. The slope of the line OA gives the Young’s modulus of the wire.
(b) Portion AB: This region is reached if the stress is increased by a very small amount. In this region, stress is not proportional to the strain. But once the stretching force is removed, the wire will regain its original length. This behaviour ends at point B and hence, the point B is known as yield point (elastic limit). The elastic hebaviour of the material (here wire) in stress-strain curve is OAB.
(c) Portion BC: If the wire is stretched beyond the point B (elastic limit), stress increases and the wire will not regain its original length after the removal of stretching force.
(d) Portion CD: With further increase in stress (beyond the point C), the strain increases rapidly and reaches the point D. Beyond D, the strain increases even when the load is removed and breaks (ruptures) at the point E. Therefore, the maximum stress (here D) beyond which the wire breaks is called breaking stress or tensile strength. The corresponding point D is known as fracture point. The region BCDE represents the plastic behaviour of the material of the wire.
Question 2.
Explain the different types of modulus of elasticity.
Answer:
From Hooke’s law, the stress in a body is proportional to the corresponding strain, provided the deformation is very small. Here we shall define the elastic modulus of a given material. There are three types of elastic modulus.
(a) Young’s modulus
(b) Rigidity modulus (or Shear modulus)
(c) Bulk modulus
Young’s Modulus: When a wire is stretched or compressed, then the ratio between tensile stress (or compressive stress) and tensile strain (or compressive strain) is defined as Young’s modules.
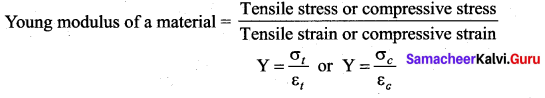
S.I. unit of Young modulus is Nm-2 or pascal.
Bulk modulus: Bulk modulus is defined as the ratio of volume stress to the volume strain.

The negative sign indicates when pressure is applied on the body, its volume decreases. Further, the equation implies that a material can be easily compressed if it has a small value of bulk modulus. In other words, bulk modulus measures the resistance of solids to change in their volume. For an example, we know that gases can be easily compressed than solids, which means, gas has a small value of bulk modulus compared to solids. The S.I. unit of K is the same as that of pressure i.e., N m– 2 or Pa (pascal).
The rigidity modulus or shear modulus: The rigidity modulus is defined as the ratio of the shearing stress to shearing strain,
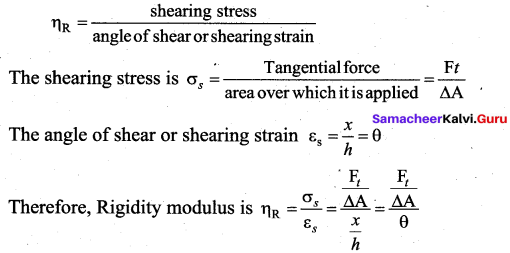
Further, the above implies, that a material can be easily twisted if it has small value of rigidity modulus. For example, consider a wire, when it is twisted through an angle θ, a restoring torque is developed, that is
\(\tau \propto \theta\)
This means that for a larger torque, wire will twist by a larger amount (angle of shear θ is large). Since, rigidity modulus is inversely proportional to angle of shear, the modulus of rigidity is small. The S.I. unit of ηR is the same as that of pressure i.e., N m-2 or pascal.
![]()
Question 3.
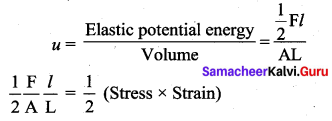
Derive an expression for the elastic energy stored per unit volume of a wire.
Answer:
When a body is stretched, work is done against the restoring force (internal force). This work done is stored in the body in the form of elastic energy. Consider a wire whose un-stretch length is L and area of cross section is A. Let a force produce an extension 1 and further assume that the elastic limit of the wire has not been exceeded and there is no loss in energy. Then, the work done by the force F is equal to the energy gained by the wire.
The work done in stretching the wire by dl, dW = Fdl
The total work done in stretching the wire from 0 to l is
![]()
From Young’s modulus of elasticity
![]()
Substituting equation (2) in equation (1), we get
![]()
Since, l is the dummy variable in the integration, we can change l to l’ (not in limits), therefore
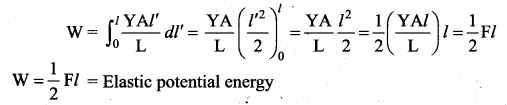
Energy per unit volume is called energy density
Question 4.
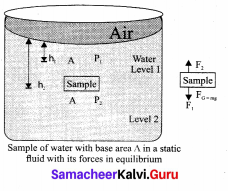
Derive an equation for the total pressure at a depth ‘A’ below the liquid surface.
Answer:
In order to understand the increase in pressure with depth below the water surface, consider a water sample of cross sectional area in the form of a cylinder. Let h1 and h2 be the depths from the air-water interface to level 1 and level 2 of the cylinder, respectively. Let F1 be the force acting downwards on level 1 and F2 be the force acting upwards on level 2, such that, F1 = P1 A and F2 = P2A. Let us assume the mass of the sample to be m and under equilibrium condition, the total upward force (F2) is balanced by the total downward force (F1 + mg), in other words, the gravitational force will act downward which is being exactly balanced by the difference between the force F2 – F1.
F2 – F1 = mg …… (1)
where m is the mass of the water available in the sample element. Let ρ be the density of the water then, the mass of water available in the sample element is
m = ρV = ρA(h2 – h1)
V = A(h2 – h1)
Hence, gravitational force, FG = ρA (h2 – h1)g
On substituting the W value in equation (1)
F2 = F1 + mg ⇒ P2A = P1A + ρA(h2 – h1)g
Cancelling out A on both sides,
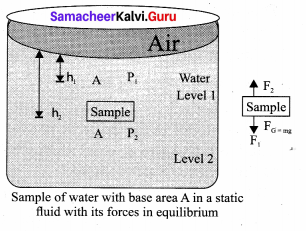
P2 = P1 + ρ(h2 – h1 )g …(2)
If we choose the level 1 at the surface of the liquid (i.e., air-water interface) and the level 2 at a depth ‘h’ below the surface, then the value of h1 becomes zero (h1 = 0) and in turn P1 assumes the value of atmospheric pressure (say Pa). In addition, the pressure (P2) at a depth becomes P. Substituting these values in equation, we get
P = Pa + ρgh …… (3)
which means, the pressure at a depth h is greater than the pressure on the surface of the liquid, where Pa is the atmospheric pressure which is equal to 1.013 × 105 Pa.
If the atmospheric pressure is neglected or ignored then P = ρgh ….. (4)
for a given liquid, ρ is fixed and g is also constant, then the pressure due to the fluid column is directly proportional to vertical distance or height of the fluid column. This implies, the height of the fluid column is more important to decide the pressure and not the cross sectional or base area or even the shape of the container.
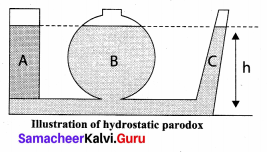
Let us consider three vessels of different shapes A, B and C as shown in figure. These vessels are connected at the bottom by a horizontal pipe. When they are filled with a liquid (say water), it occupies the same level even though the vessels hold different amounts of water. It is true because the liquid at the bottom of each section of the vessel experiences the same pressure.
![]()
Question 5.
State and prove Pascal’s law in fluids.
Answer:
Statement of Pascal’s law: If the pressure in a liquid is changed at a particular point, the change is transmitted to the entire liquid without being diminished in magnitude.

Application of Pascal’s law: Hydraulic lift: A practical application of Pascal’s law is the hydraulic lift which is used to lift a heavy load with a small force. It is a force Hydraulic lift multiplier. In consists of two cylinders A and B connected to each other by a horizontal pipe, filled with a liquid. They are fitted with frictionless pistons of cross sectional areas A1 and A2 (A2 > A1). Suppose a downward force F is applied on the smaller piston, the pressure of the liquid under this piston increase to 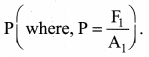 But according to Pascal’s law, this increased pressure P is transmitted undiminished in all directions. So a pressure is exerted on piston B. Upward force on piston B is
But according to Pascal’s law, this increased pressure P is transmitted undiminished in all directions. So a pressure is exerted on piston B. Upward force on piston B is
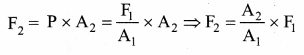
Therefore by changing the force on the smaller piston A, the force on the piston B has been increased by the factor \(\frac{\mathrm{A}_{2}}{\mathrm{A}_{1}}\) and this factor is called the mechanical advantage of the lift.
Question 6.
State and prove Archimedes principle.
Answer:
Archimedes Principle: It states that when a body is partially or wholly immersed in a fluid, it experiences an upward thrust equal to the weight of the fluid displaced by it and its upthrust acts through the centre of gravity of the liquid displaced.
Upthrust (or) buoyant force = Weight of liquid displaced
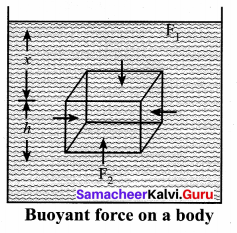
Proof: Consider a body of height ‘h’ lying inside a liquid of density ρ, at a depth x below the free surface of the liquid. Area of cross section of the body is ‘a’. The forces on the sides of the body cancel out.
Pressure at the upper face of the body, P1 = xρg
Pressure at the lower face of the body, P2 = (x + h)ρg
Thrust acting on the upper face of the body is F1 = P1a = xρga acting vertically downwards,
Thrust acting on the lower face of the body is F2 = P2a = (x + h) ρga acting vertically upwards.
The resultant force (F2 – F1) is acting on the body direction and is called upthrust (U).
U = F2 – F1 = (x + h) ρga – xρga = ahρg
But ah = V, Volume of the body = Volume of liquid
U = Vρg = Mg
i.e., Upthrust or buoyant force = Weight of liquid displaced.
This proves the Archimedes principle.
Question 7.
Derive the expression for the terminal velocity of a sphere moving in a high viscous fluid using stokes force.
Answer:
Expression for terminal velocity: Consider a sphere of radius r which falls freely through a
highly viscous liquid of coefficient of viscosity η. Let the density of the material of the sphere be ρ and the density of the fluid be σ.
Gravitational force acting on the sphere,

Here, it should be noted that the terminal speed of the sphere is directly proportional to the
square of its radius. If a is greater than ρ, then the term (ρ – σ) becomes negative leading to a
negative terminal velocity.
![]()
Question 8.
Derive Poiseuille’s formula for the volume of a liquid flowing per second through a pipe
under streamlined flow.
Answer:
Consider a liquid flowing steadily through a horizontal capillary tube. Let v = \(\left(\frac{\mathrm{v}}{t}\right)\) be the volume of the liquid flowing out per second through a capillary tube. It depends on (1) coefficient of viscosity (η) of the liquid, (2) radius of the tube (r), and (3) the pressure gradient \(\left(\frac{P}{l}\right)\).
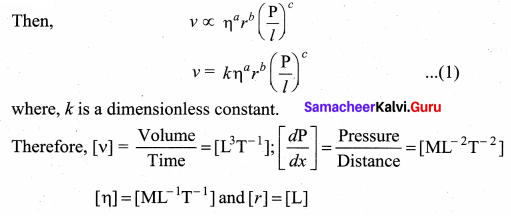
Substituting in equation (1)
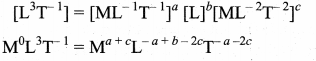
So, equating the powers of M, L and T on both sides, we get
a + c = 0, -a + b – 2c = 3, and – a – 2c = – 1
We have three unknowns a, b and c. We have three equations, on solving, we get
a = – 1, b = 4 and c = 1
Therefore, equation (1) becomes,

Experimentally, the value of k is shown to be \(\frac{\pi}{8}\), we have

The above equation is known as Poiseuille’s equation for the flow of liquid through a narrow tube or a capillary tube. This relation holds good for the fluids whose velocities are lesser than the critical velocity (vc).
Question 9.
Obtain an expression for the excess of pressure inside a
(i) liquid drop
(ii) liquid bubble
(iii) air bubble.
Answer:
1. Excess of pressure inside air bubble in a liquid:
Consider an air bubble of radius R inside a liquid having surface tension T. Let P1 and P2 be the pressures outside and inside the air bubble, respectively.
Now, the excess pressure inside the air bubble is ∆P = P1 – P2
In order to find the excess pressure inside the air bubble, let us consider the forces acting on the air bubble. For the hemispherical portion of the bubble, considering the forces acting on it, we get,
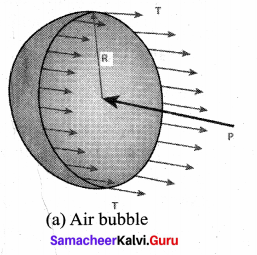
(i) The force due to surface tension acting towards right around the rim of length 2πR is FT = 2πRT
(ii) The force due to outside pressure P, is to the right acting across a cross sectional area of πR2 is \(\mathrm{P}_{1} \pi \mathrm{R}^{2}\)
(iii) The force due to pressure P2 inside the bubble, acting to the left is \(\mathrm{F}_{\mathrm{P}_{2}}=\mathrm{P}_{2} \pi \mathrm{R}^{2}\)
As the air bubble is in equilibrium under the action of these forces, \(\mathrm{F}_{\mathrm{P}_{2}}=\mathrm{F}_{\mathrm{T}}+\mathrm{F}_{\mathrm{P}_{1}}\)
Excess pressure is ∆P = P2 – P1 = \(\frac{2 \mathrm{T}}{\mathrm{R}}\)
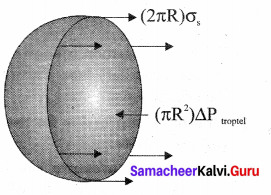
2. Excess pressure inside a soap bubble: Consider a soap bubble of radius R and the surface tension of the soap bubble be T. A soap bubble has two liquid surfaces in contact with air, one inside the bubble and other outside the bubble. Therefore, the force on the soap bubble due to surface tension is 2 × 2πRT. The various forces acting on the soap bubble are,

(i) Force due to surface tension FT = 4πRT towards right.

3. Excess pressure inside the liquid drop: Consider a liquid drop of radius R and the surface tension of the liquid is T.
The various forces acting on the liquid drop are:

![]()
Question 10.
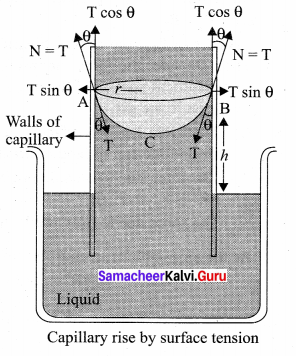
What is capillarity? Obtain an expression for the surface tension of a liquid by capillary rise method.
Answer:
In a liquid whose angle of contact with solid is less than 90°, suffers capillary rise. On the other hand, in a liquid whose angle of contact is greater than 90°, suffers capillary fall. The rise or fall of a liquid in a narrow tube is called capillarity or capillary action.
Practical application of capillarity
(i) Due to capillary action, oil rises in the cotton within an earthen lamp. Likewise, sap raises from the roots of a plant to its leaves and branches.
(ii) Absorption of ink by a blotting paper.
(iii) Capillary action is also essential for the tear fluid from the eye to drain constantly.
(iv) Cotton dresses are preferred in summer because cotton dresses have fine pores which act as capillaries for sweat.
Surface Tension by capillary rise method: The pressure difference across a curved liquid-air interface is the basic factor behind the rising up of water in a narrow tube (influence of gravity is ignored). The capillary rise is more dominant in the case of very fine tubes. But this phenomenon is the outcome of the force of surface tension. In order to arrive a relation between the capillary rise (h) and surface tension (T), consider a capillary tube which is held vertically in a beaker containing water, the water rises in the capillary tube to a height h due to surface tension.
The surface tension force FT, acts along the tangent at the point of contact downwards and its reaction force upwards. Surface tension T, it resolved into two components
(i) Horizontal component T sin θ and
(ii) Vertical component T cos θ acting upwards, all along the whole circumference of the meniscus Total upward force = (T cos θ) (2πr) = 2πrT cos θ
where θ is the angle of contact, r is the radius of the tube. Let ρ be the density of water and h be the height to which the liquid rises inside the tube. Then,
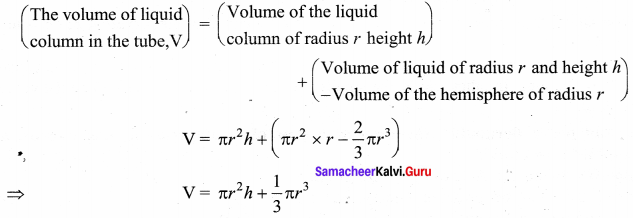
The upward force supports the weight of the liquid column above the free surface, therefore,
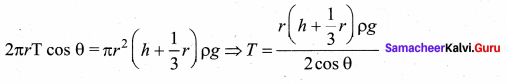
If the capillary is a very fine tube of radius (i.e., radius is very small) then \(\frac{r}{3}\) can be neglected when it is compared to the height h. Therefore,
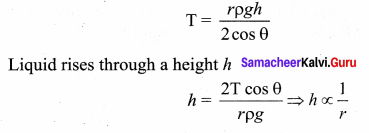
This implies that the capillary rise (h) is inversely proportional to the radius (r) of the tube, i. e., the smaller the radius of the tube greater will be the capillarity.
Question 11.
Obtain an equation of continuity for a flow of fluid on the basis of conservation of mass.
Answer:
The mass flow rate through a pipe, it is necessary to assume that the flow of fluid is steady, the flow of the fluid is said to be steady if at any given point, the velocity of each passing fluid particle remains constant with respect to time.

Under this condition, the path taken by the fluid particle is a streamline.
Consider a pipe AB of varying cross sectional area a1 and a2 such that a1 > a2. A non-viscous -and incompressible liquid flows steadily through the pipe, with velocities v1 and v2 in area a1 and a2, respectively.
Let m1 be the mass of fluid flowing through section A in time ∆t, m1 = (a1v1∆t)ρ
Let m2 be the mass of fluid flowing through section B in time ∆t, m2 = (a2v2∆t)ρ
For an incompressible liquid, mass is conserved m1 = m2
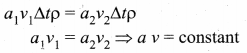
which is called the equation of continuity and it is a statement of conservation of mass in the flow of fluids.
In general, a v = constant, which means that the volume flux or flow rate remains constant throughout the pipe. In other words, the smaller the cross section, greater will be the velocity of the fluid.
Question 12.
State and prove Bernoulli’s theorem for a flow of incompressible, non-viscous, and streamlined flow of fluid.
Answer:
Bernoulli’s theorem: According to Bernoulli’s theorem, the sum of pressure energy, kinetic energy, and potential energy per unit mass of an incompressible, non-viscous fluid in a streamlined flow remains a constant. Mathematically,
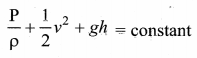
This is known as Bernoulli’s equation.
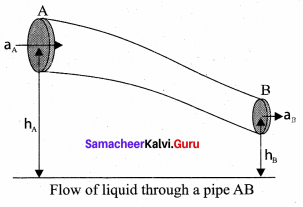
Proof: Let us consider a flow of liquid through a Flow of liquid through a pipe AB
pipe AB. Let V be the volume of the liquid when it enters A in a time t which is equal to the volume of the liquid leaving B in the same time. Let aA, vA and PA be the area of cross section of the tube, velocity of the liquid and pressure exerted by the liquid at A respectively.
Let the force exerted by the liquid at A is FA = PAaA
Distance travelled by the liquid in time t is d = vAt
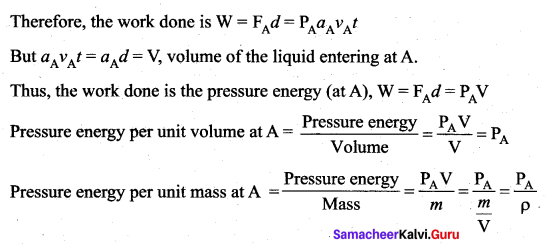
since m is the mass of the liquid entering at A in a given time, therefore, pressure energy of the liquid at A is
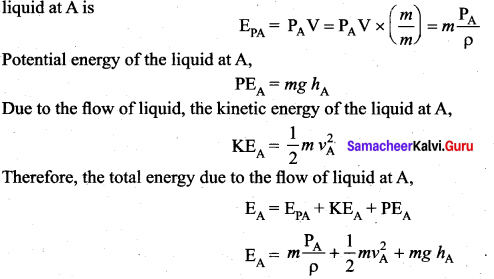
Similarly, let aB, vB and PB be the area of cross section of the tube, velocity of the liquid and pressure exerted by the liquid at B. Calculating the total energy at EB, we get
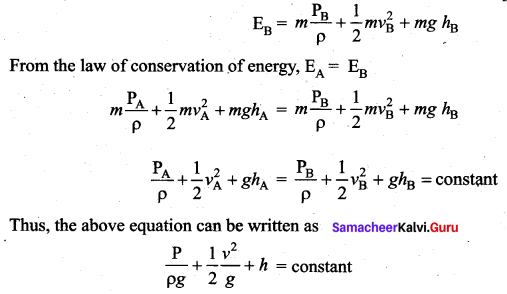
The above equation is the consequence of the conservation of energy which is true until there is no loss of energy due to friction. But in practice, some energy is lost due to friction. This arises due to the fact that in a fluid flow, the layers flowing with different velocities exert frictional forces on each other. This loss of energy is generally converted into heat energy. Therefore, Bernoulli’s relation is strictly valid for fluids with zero viscosity or non-viscous liquids. Notice that when the liquid flows through a horizontal pipe, then
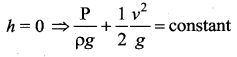
Question 13.
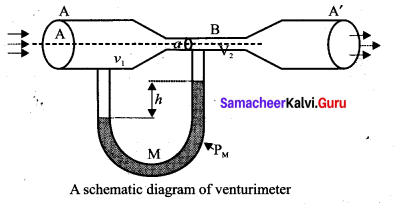
Describe the construction and working of venturimeter and obtain an equation for the volume of liquid flowing per second through a wider entry of the tube.
Answer:
Venturimeter : This device is used to measure the rate of flow (or say flow speed) of the incompressible fluid flowing through a pipe. It works on the principle of Bernoulli’s theorem. It consists of two wider tubes A and A’ (with cross sectional area A) connected by a narrow tube B (with cross sectional area a). A manometer in the form of U-tube is also attached between the wide and narrow tubes. The manometer contains a liquid of density ‘ρm’.
Let P1 be the pressure of the fluid at the wider region of the tube A. Let us assume that the fluid of density ‘ρ’ flows from the pipe with speed ‘v1’ and into the narrow region, its speed increases to ‘v2’. According to the Bernoulli’s equation, this increase in speed is accompanied by a decrease in the fluid pressure P2 at the narrow region of the tube B. Therefore, the pressure difference between the tubes A and B‘ is noted by measuring the height difference (∆P = P1 – P2) between the surfaces of the manometer liquid.
From the equation of continuity, we can say that Av1 = av2 which means that

From the above equation, the pressure difference

The volume of the liquid flowing out per second is
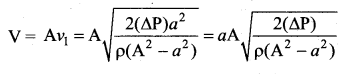
Samacheer Kalvi 11th Physics Properties of Matter Numerical Problems
Question 1.
A capillary of diameter dmm is dipped in water such that the water rises to a height of 30 mm. If the radius of the capillary is made of its previous value, then compute the
height up to which water will rise in the new capillary?
Answer:
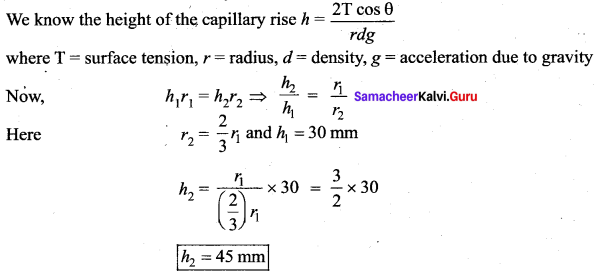
Question 2.
A cylinder of length 1.5 m and diameter 4 cm is fixed at one end. A tangential force of 4 × 105 N is applied at the other end. If the rigidity modulus of the cylinder is 6 × 1010 Nm-2 then, calculate the twist produced in the cylinder.
Answer:
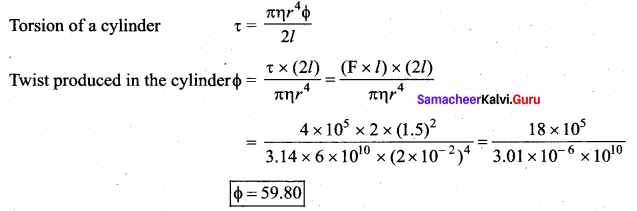
Question 3.
A spherical soap bubble A of radius 2 cm is formed inside another bubble B of radius 4 cm. Show that the radius of a single soap bubble which maintains the same pressure difference as inside the smaller and outside the larger soap bubble is lesser than radius of both soap bubbles A and B.
Answer:
From the excess pressure inside a soap bubble

Here the two bubbles having the same pressure and temperature. So the radius of the combined bubbles,
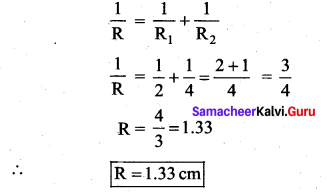
![]()
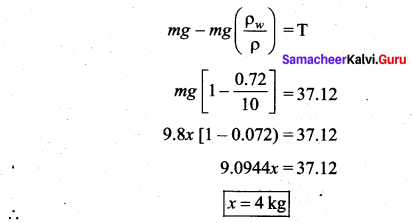
Question 4.
A block of Ag of mass x kg hanging from a string is immersed in a liquid of relative density 0.72. If the relative density of Ag is 10 and tension in the string is 37.12 N then compute the mass of Ag block.
Answer:
From the terminal velocity condition, FG – U = F F = T, m = x
Question 5.
The reading of pressure meter attached with a closed pipe is 5 × 105 Nm– 2. On opening the valve of the pipe, the reading of the pressure meter is 4.5 × 105 Nm-2. Calculate the speed of the water flowing in the pipe.
Answer:
Using Bernoulli’s equation
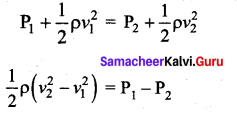
Here initial velocity V1 = 0 and density of water p = 1000 kg m-3
![]()
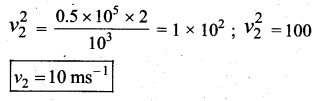
Samacheer Kalvi 11th Physics Properties of Matter Conceptual Questions
Question 1.
Why coffee runs up into a sugar lump (a small cube of sugar) when one corner of the sugar lump is held in the liquid?
Answer:
Dip the comer of a sugar cube in coffee, and get the whole cube coffee-flavoured due to “capillary action”.
Question 2.
Why two holes are made to empty an oil tin?
Answer:
When oil comes out through a tin with one hole, the pressure inside the tin becomes less than the atmospheric pressure, soon the oil stops flowing out. When two holes are made in the tin, air keeps on entering the tin through the other hole and maintains pressure inside.
Question 3.
We can cut vegetables easily with a sharp knife as compared to a blunt knife. Why?
Answer:
The area of a sharp edge is much less than the area of a blunt edge. For the same total force, the effective force per unit area is more for the sharp edge than the blunt edge. Hence, a sharp knife cuts easily than a blunt knife.
Question 4.
Why the passengers are advised to remove the ink from their pens while going up in an aeroplane?
Answer:
We know that atmospheric pressure decreases with height. Since ink inside the pen is filled at the atmospheric pressure existing on the surface of Earth, it tends to come out to equalise the pressure. This can spoil the clothes of the passengers, so they are advised to remove the ink from the pen.
Question 5.
We use straw to suck soft drinks, why?
Answer:
When we suck through the straw, the pressure inside the straw becomes less than the atmospheric pressure. Due to the pressure difference, the soft drink rises in the straw and we are able to take the soft drink easily.
Samacheer Kalvi 11th Physics Properties of Matter Additional Questions
I. Choose the correct answer from the following:
Question 1.
The force required to stretch a steel wire 1 cm2 in cross section to double its length is (given Y = 2 × 1011 Nm-2) ……….
![]()
Answer:
(b) 2 × 107 N
Solution:
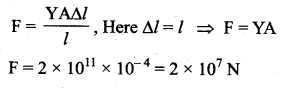
Question 2.
The fractional change in volume per unit increase in pressure is called ……..
(a) Pressure co-efficient
(b) Volume co-efficient
(c) Bulk modulus
(d) Compressibility
Answer:
(d) Compressibility
Question 3.
The modulus of rigidity of a liquid is
(a) zero
(b) 1
(c) infinite
(d) none of these
Answer:
(a) zero
![]()
Question 4.
The Young’s modulus of a wire of length L and radius r is Y. If the length is reduced to \(\frac{\mathrm{L}}{2}\) and radius to \(\frac{\mathrm{r}}{2}\), its Young’s modulus will be …….
(a) \(\frac{\mathrm{Y}}{2}\)
(b) Y
(c) 2Y
(d) 4Y
Answer:
(b) Y
Solution:
The Young’s modulus is a property of the material. So, it remains the same.
Question 5.
A spherical ball contracts in volume by 0.01% when subjected to a normal uniform pressure of 100 atmospheres. The bulk modulus of the material of the ball in dynes/cm2 is …….
(a) 1 × 1012
(b) 10 × 1012
(c) 100 × 1012
(d) 2 × 1011
Answer:
(a) 1 × 1012
Solution:

Question 6.
The value of Poisson’s ratio lies between
(a) 0 and 1
(b) – 0.5 and 1
(c) 0 and 0.5
(d) – 1 and 1
Answer:
(c) 0 and 0.5
![]()
Question 7.
Poisson’s ratio cannot have the value ……
(a) 0.1
(b) 0.2
(c) 0.5
(d) 0.7
Answer:
(d) 0.7
Question 8.
The bulk modulus for an incompressible fluid is ………
(a) zero
(b) 1
(c) ∞
(d) between 0 and 1
Answer:
(c) ∞
Question 9.
The breaking stress of a wire depends upon
(a) length of the wire
(b) material of the wire
(c) radius of the wire
(d) shape of the cross-section
Answer:
(b) material of the wire
Question 10.
Shearing stress causes change in ……
(a) length
(b) breadth
(c) shape
(d) volume
Answer:
(c) shape
Question 11.
A certain force increases the length of a wire by 1 mm. The force required to increases its length by 2 mm is …….
(a) 2F
(b) 4F
(c) 8F
(d) 16F
Answer:
(a) 2F
Solution:
∆l ∝ F
![]()
Question 12.
Two wires of same material, having cross-sectional areas in the ratio 1 : 2 and lengths in the ratio 1 : 4 are stretched by the same force. The ratio of the stresses in the wires will be …….
(a) 1 : 2
(b) 2 : 1
(c) 1 : 4
(d) 4 : 1
Answer:
(b) 2 : 1
Solution:
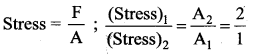
Question 13.
If the tension on a wire is removed at once, then ……..
(a) it will break
(b) its temperature will reduce
(c) there will be no change in its temperature
(d) its temperature will increase
Answer:
(d) its temperature will increase
Solution:
When tension is applied, the distance between the atoms of the wire increases, there by increasing the potential energy of the wire. When the tension is removed, the potential energy decreases. This energy is converted into heat energy. So the temperature of the wire increases.
Question 14.
In steel, the Young’s modulus and the strain at the breaking point are 2 × 1011 Nm-2 and 0.15 respectively. The stress at the breaking point for steel is therefore ………
(a) 2 × 108 Nm-2
(b) 3 × 1010 Nm-2
(c) 3 × 1012Nm-2
(d) None of these
Answer:
(b) 3 × 1010 Nm-2
Solution:
Breaking stress = Breaking strain × Young’s modulus
= 0.15 × 2 × 1011 = 3 × 1010 Nm-2 .
Question 15.
The pressure in a liquid at a given depth below the surface ……….
(a) is always exerted downward
(b) is the same in all directions
(c) equals the total weight of liquids above that depth
(d) depends upon the amount of liquid below that depth
Answer:
(b) is the same in all directions
Question 16.
The pressure at the bottom of a liquid tank does not depend on ………
(a) acceleration due to gravity
(b) density of the liquid
(c) height of the liquid
(d) area of the liquid surface
Answer:
(d) area of the liquid surface
Question 17.
The pressure of the Earth’s atmosphere at sea level is due to the ……
(a) gravitational attraction of the Earth for the atmosphere
(b) evaporation of water from the seas and oceans
(c) fact that most living things constantly breathe air
(d) heating of the atmosphere by the Sun
Answer:
(a) gravitational attraction of the Earth for the atmosphere
![]()
Question 18.
The operating principle of a hydraulic press is …….
(a) Pascal’s Law
(b) Archimedes principle
(c) Newton’s law of gravitation
(d) Boyle’s law
Answer:
(a) Pascal’s Law
Question 19.
A floating body always displaces its own ……..
(a) mass of liquid
(b) volume of liquid
(c) weight of liquid
(d) none of these
Answer:
(c) weight of liquid
Question 20.
The pressure in a water tap at the base of a building is 3 × 106 dynes/cm2 and on its top it is 1.6 × 106 dynes/cm2. The height of the building is approximately
(a) 7 m
(b) 14 m
(c) 70 m
(d) 140 m
Answer:
(b) 14 m
Solution:
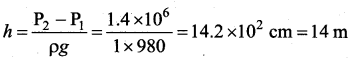
Question 21.
The weight of a body in air is 100 N. How much will it weight in water, if it displaces 400 cc of water?
(a) 90 N
(b) 94 N
(c) 98 N
(d) None of these
Answer:
(d) None of these
Solution:
Upthrust = Weight of water displaced = 0.4 × 9.8 = 3.92 N
Apparent weight = 100 – 3.92 = 96.08 N
Question 22.
A body is floating in a liquid with \(\frac{1}{5}\) of its volume outside the liquid. If the relative density of the body is 0.9, that of the liquid is ……..
![]()
Answer:
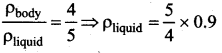
Question 23.
A boat having length 3m and breadth 2m is floating on a lake. It sinks by 1 cm when a man gets on it. The mass of the man is ………
(a) 60 kg
(b) 55 kg
(c) 65 kg
(d) 70 kg
Answer:
(a) 60kg
Solution:
Weight of man = Weight of additional water displaced
mg = 3 × 2 × 0.01 × 103 × g m = 60 kg
Question 24.
A bird weighs 2 kg and is inside an airtight cage of 1 kg. If its starts to fly, then what is the weight of the bird and cage assembly?
(a) 3 kg
(b) 2 kg
(c) 1 kg
(d) none of these
Answer:
(a) 3 kg
Solution:
When the bird flies, the upthrust on it is equal and opposite to the down thrust on the cage. Therefore, the weight of the assembly remains unchanged.
Question 25.
Two light balls are suspended as shown in the figure. When a stream of air passes through the space between them, the distance between the balls will ……..
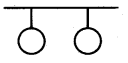
(a) increase
(b) decrease
(c) remain the same
(d) may increase or decrease depending on the speed of air
Answer:
(b) decrease
Solution:
When the speed of the air between the balls increases, then according to Bernoulli’s theorem, the pressure in this region decreases. Therefore, the balls will be pushed towards each other by the air pressure in the outer region.
Question 26.
The rate of leak from a hole in a tank is …….
(a) independent of its height from the bottom
(b) more if situated near the bottom
(c) more if situated near its top
(d) more at midway between top and bottom
Answer:
(b) more if situated near the bottom
Question 27.
When a fluid passes through the constricted part of a pipe, its ………
(a) velocity and pressure decrease
(b) velocity and pressure increase
(c) velocity decreases and pressure increases
(d) velocity increases and pressure decreases
Answer:
(d) velocity increases and pressure decreases
Question 28.
Bernoulli’s principle does not explain ……….
(a) curved path of a spinning ball
(b) surviving of a fish in a lake
(c) working of a paint sprayer
(d) automatic blowing off the roofs of houses during blizzard in hilly areas
Answer:
(b) surviving of a fish in a lake
Question 29.
An ideal liquid flows through a horizontal tube of variable diameter. The pressure is lowest where the ……..
(a) velocity is highest
(b) velocity is lowest
(c) diameter is largest
(d) none of these
Answer:
(a) velocity is highest
Question 30.
Bernoulli’s equation is applicable in the case of ……….
(a) streamlined flow of compressible fluids
(b) streamlined flow of incompressible fluids
(c) turbulent flow of compressible fluids
(d) turbulent flow of incompressible fluids
Answer:
(b) streamlined flow of incompressible fluids
Question 31.
Bernoulli’s theorem is based on the conservation of …….
(a) mass
(b) momentum
(c) energy
(d) all of the above
Answer:
(c) energy
![]()
Question 32.
Bernoulli’s theorem is applicable to ………
(a) flow of liquids
(b) viscocity
(c) surface tension
(d) static fluid pressure
Answer:
(a) flow of liquids
Question 33.
The working of an atomiser depends on ….
(a) Bernoulli’s principle
(b) Boyle’s law
(c) Archimedes principle
(d) Pascal’s law
Answer:
(a) Bernoulli’s principle
Question 34.
‘Dynamic lift’ is related to ……
(a) Bernoulli’s principle
(b) Archimedes principle
(c) Equation of continuity
(d) Pascal’s law
Answer:
(a) Bernoulli’s principle
Question 35.
A gale blows over a house. The force due to the gale on the roof is ………
(a) in the downward direction
(b) zero
(c) in the upward direction
(d) horizontal
Answer:
(c) in the upward direction
Question 36.
If a stream of air is blown under one of the pans of a physical balance in equilibrium, then the
pan will ……..
(a) go up
(b) go down
(c) not be affected
(d) go up or down depending on the velocity of the stream
Answer:
(b) go down
Question 37.
Water venturimeter works on the principle of ……….
(a) Newton’s third law of motion
(b) Stokes’s formula
(c) Bernoulli’s theorem
(d) Hooke’s law
Answer:
(c) Bernoulli’s theorem
Question 38.
Aeroplanes are made to run on runway before take-off because it ………
(a) decreases friction
(b) decreases viscous drag of air
(c) decreases atmospheric pressure
(d) provides required life to the aeroplane
Answer:
(d) provides required life to the aeroplane
Question 39.
When the terminal velocity is reached, the acceleration of a body moving through a viscous
medium is …….
(a) zero
(b) positive
(c) negative
(d) either (b) or (c) depending upon other factors.
Answer:
(a) zero
Question 40.
If a raindrop with a mass of 0.05 g falls with constant velocity, the retarding force of atmospheric
friction is (neglect density of air) …….
(a) zero
(b) 49 dynes
(c) 490 dynes
(d) none of these
Answer:
(b) 49 dynes
Solution:
Since the rain drop is falling with constant velocity, the retarding upward force is equal to its weight in magnitude, F = 0.05 × 980 = 49 dynes
Question 41.
If temperature rises, the coefficient of viscosity of a liquid …….
(a) decreases
(b) increases
(c) remains unchanged
(d) increases for some liquids and decreases for others
Answer:
(a) decreases
![]()
Question 42.
The velocity of a rain drop attains constant value because of ……..
(a) surface tension
(b) upthrust of air
(c) viscous force exerted by air
(d) air currents
Answer:
(c) viscous force exerted by air
Question 43.
With increase in temperature the viscosity of
(a) a gas decreases and a liquid increases
(b) a gas increases and a liquid decreases
(c) both gases and liquids decrease
(d) both gases and liquids increase
Answer:
(b) a gas increases and a liquid decreases
Question 44.
Two small spheres of radii r and 2r fall through a viscous liquid with the same constant speed.
The viscous forces experienced by them are in the ratio ……..
(a) 1 : 2
(b) 2 : 1
(c) 1 : 4
(d) 4 : 1
Answer:
(a) 1 : 2
Solution:
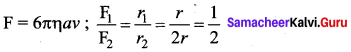
Question 45.
Viscosity is the property of liquids by virtue of which they ……
(a) oppose the relative motion of its parts
(b) push neighbouring molecules
(c) attract other molecules
(d) become conducting
Answer:
(a) oppose the relative motion of its parts
Question 46.
Streamlined flow is more likely for liquids with ………
(a) high density and low viscosity
(b) low density and high viscosity
(c) high density and high viscosity
(d) low density and low viscosity
Answer:
(b) low density and high viscosity
Question 47.
The dimensional formula of coefficient of viscosity is ……
![]()
Answer:
(d) ML-1 T-1
Question 48.
A good lubricant should have …….
(a) high viscosity
(b) low viscosity
(c) moderate viscosity
(d) high density
Answer:
(a) high viscosity
![]()
Question 49.
If a liquid wets a solid surface, the angle of contact is …….
(a) 0°
(b) 90°
(c) less than 90°
(d) greater than 90°
Answer:
(c) less than 90°
Question 50.
When some detergent is added to water, the surface tension ……..
(a) remains unaffected
(b) increases
(c) decreases
(d) may increase or decrease
Answer:
(c) decreases
Question 51.
Rain drops are spherical because of …….
(a) gravitational force
(b) surface tension
(c) low viscosity of water
(d) air resistance
Answer:
(b) surface tension
Question 52.
At critical temperature the surface tension of a liquid …….
(a) is zero
(b) is the same as that at any other temperature
(c) is infinity
(d) cannot be determined
Answer:
(a) is zero
Question 53.
A liquid will not wet the surface of a solid if the angle of contact is …..
(a) acute
(b) obtuse
(c) zero
(d) \(\frac{\pi}{2}\)
Answer:
(b) obtuse
Question 54.
The surface tension of soap solution is 25 × 10-3 Nm-1. The excess pressure inside a soap bubble of diameter 1 cm is ………
(a) 5 Pa
(b) 10 Pa
(c) 20 Pa
(d) None of these
Answer:
(c) 20 Pa
Solution:
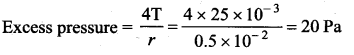
Question 55.
Surface tension does not depend on ……..
(a) nature of the liquid
(b) temperature of the liquid
(c) atmospheric pressure
(d) presence of impurities
Answer:
(c) atmospheric pressure
Question 56.
Meniscus of mercury in a capillary is ……
(a) concave
(b) convex
(c) plane
(d) cylindrical
Answer:
(b) convex
![]()
Question 57.
The potential energy of a molecule on the surface of a liquid compared to that of a molecules
inside the liquid is …….
(a) smaller
(b) the same
(c) greater
(d) zero
Answer:
(c) greater
Question 58.
At which of the following temperatures, the value of surface tension of water is minimum?
(a) 4°C
(b) 25°C
(c) 50°C
(d) 15°C
Answer:
(d) 15°C
Question 59.
If the surface tension of water is 0.06 Nm-1 then the capillary rise in a tube of diameter 1 mm is (angle of contact = 0°) ……..
(a) 1.22 cm
(b) 2.44 cm
(c) 3.12 cm
(d) 3.86 cm
Answer:
(b) 2.44 cm
Solution:

Question 60.
The surface tension phenomenon is the result of the tendency of a system to ………
(a) conserve energy
(b) conserve volume
(c) keep potential energy minimum
(d) keep surface area minimum
Answer:
(c) keep potential energy minimum
Samacheer Kalvi 11th Physics Properties of Matter 2 Mark Questions
II. Write brief answer to the following questions:
Question 1.
What is meant by ‘Mean positions of atoms’?
Answer:
The interaction between the atoms, they position themselves at a particular interatomic distance. This position of atoms in this bound condition is called their mean positions.
Question 2.
What is meant by ‘Evaporation’?
Answer:
When a liquid is heated at constant pressure to its boiling point or when the pressure is reduced at a constant temperature it will convert to a gas. This process of a liquid changing to a gas is called evaporation.
Question 3.
What are the physical states of matter?
Answer:
Solid, liquid, gas, plasma, Bose-Einstein condensates, quark-gluon plasmas and hot plasma these are the physical states of matter.
Question 4.
Define elasticity? Give its example.
Answer:
A body regains its original shape and size after the removal of deforming force, it is said be elastic and the property is called elasticity. Example: Rubber, metals, steet ropes
Question 5.
Define deforming force?
Answer:
The force which changes the size or shape of a body is called a deforming force.
Question 6.
Define ‘Plasticity’?
Answer:
If a body does not regain its original shape and size after removal of the deforming force, it is said to be a plastic body and the property is called plasticity.
Example : Glass.
![]()
Question 7.
Explain the classification of longitudinal stress?
Answer:
Longitudinal stress can be classified into two types, tensile stress and compressive stress. Tensile stress: Internal forces on the two sides of ∆A may pull each other, i.e., it is stretched by equal and opposite forces. Then, the longitudinal stress is called tensile stress. Compressive stress: When forces acting on the two sides of ∆A push each other, ∆A is pushed by equal and opposite forces at the two ends. In this case, ∆A is said to be under compression. Then, the longitudinal stress is called compressive stress.
Question 8.
Explain the classification of longitudinal strain?
Answer:
Longitudinal strain can be classified into two types: –
- Tensile strain: If the length is increased from its natural length then it is known as tensile
strain. - Compressive strain: If the length is decreased from its natural length then it is known as compressive strain.
Question 9.
Define elastic limit?
Answer:
Elastic limit: The maximum stress within which the body regains its original size and shape after the removal of deforming force is called the elastic limit.
Question 10.
What is meant by “Breaking stress or tensile strength”?
Answer:
The maximum stress ulitimate stress point beyond which the wire breaks is called breaking stress or tensile strength.
Question 11.
Define compressibility?
Answer:
The reciprocal of the bulk modulus is called compressibility. It is defined as the fractional change in volume per unit increase in pressure.
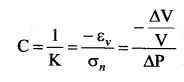
Question 12.
Define relative density (or) specific gravity?
Answer:
The relative density of a substance is defined as the ratio of the density of a substance to the density of water at 4°C. It is a dimensionless positive scalar quantity.
![]()
Question 13.
What is atmospheric pressure?
Answer:
The pressure exerted by the atmosphere at sea level is called atmospheric pressure. The atmospheric pressure at sea level is 1.013 × 105 Nm-2 (or) Pa
Question 14.
Explain hydrostatic paradox with suitable example.
Answer:
Hydrostatic paradox: The pressure exerted by a liquid column depends only on the height of the liquid column and not on the shape of the containing vessel:
Let us consider three vessels A, B and C of different shapes. These vessels are connected at the bottom by a horizontal pipe. When they are filled a liquid (say water), it occupies the same level even though the vessels hold different amount of water. It is true because the liquid at the bottom of each section of the vessel experiences the same pressure.
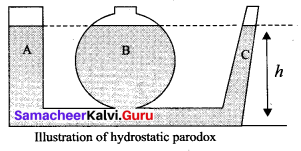
Question 15.
Write the examples of floating bodies?
Answer:
- A person can swim in sea water more easily than in river water.
- Ice floats on water.
- The ship is made of steel but its interior is made hollow by giving it a concave shape.
Question 16.
Define ‘Viscosity’?
Answer:
Viscosity is defined as ‘the property of a fluid to oppose the relative motion between its layers’.
Question 17.
Define tube of flow.
Answer:
A bundle of streamlines having the same velocity over any cross section perpendicular to the
direction of flow then such bundle is called a ‘tube of flow’.
Question 18.
Write down the applications of viscosity?
Answer:
(i) The oil used as a lubricant for heavy machinery parts should have a high viscous coefficient. To select a suitable lubricant, we should know its viscosity and how it varies with temperature. [Note: As temperature increases, the viscosity of the liquid decreases]. Also, it helps to choose oils with low viscosity used in car engines (light machinery).
(ii) The highly viscous liquid is used to damp the motion of some instruments and is used as brake oil in hydraulic brakes.
(iii) Blood circulation through arteries and veins depends upon the viscosity of fluids.
(iv) Millikan conducted the oil drop experiment to determine the charge of an electron. He used the knowledge of viscosity to determine the charge.
Question 19.
What is meant by‘Molecular range’?
Answer:
It is the maximum distance upto which a molecule can exert force of attraction on another molecule. It is of the order of 10-9 m for solids and liquids.
Question 20.
What is sphere of influence?
Answer:
It is a sphere drawn around a particular molecule as centre and molecular range as radius.
Samacheer Kalvi 11th Physics Properties of Matter Numerical Questions
Question 1.
Two steel wires of lengths 1 m and 2 m have diameters 1 mm and 2 mm respectively. If they are stretched by forces of 40 N and 80 N respectively, find the ratio of their elongations.
Solution:
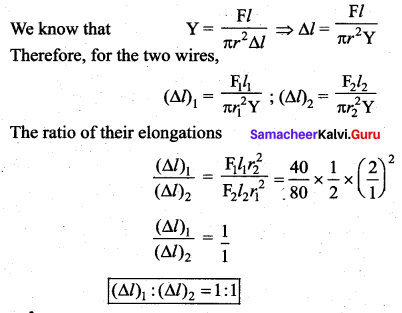
Question 2.
A wire of length 2m and cross-sectional area 2 × 10-6 m2 is made of a material of Young’s modulus 2 × 1011 Nm-2. What is the work done in stretching it through 0.1 mm.
Answer:

Question 3.
A wire is stretched by 0.01 m when it is stretched by a certain force. Another wire of the same material but double the length and double the diameter is stretched by the same force. What is the elongation in metres?
Answer:
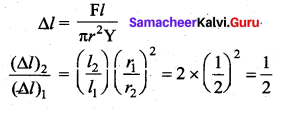
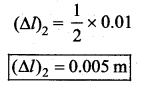
Question 4.
Two wires of same material and same diameter have lengths in the ratio 2 : 5. They are stretched by same force. Calculate the ratio of workdone in stretching them.
Answer:
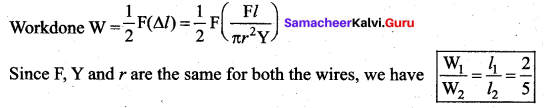
Question 5.
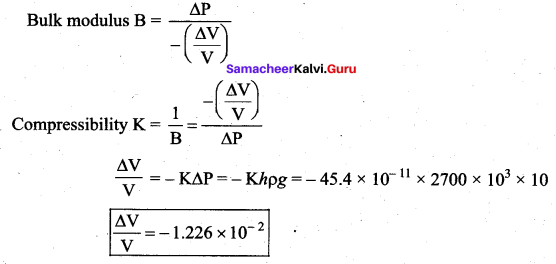
The approximate depth of an ocean is 2700 m. The compressibility of water is 45.4 × 10-11 Pa-1 and density of water is 103 kg m-3. What fractional compression of water will be obtained at the bottom of the ocean?
Answer:
Question 6.
An iceberg of density 900 kgm-3 is floating in water of density 1000 kgm-3. What is the percentage of volume of iceberg outside the water?
Answer:
Fraction of volume inside water = Relative density of the body
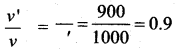
Fraction of volume outside water = 1 – 0.9 = 0.1
Percentage of volume outside water = 0.1 × 100 = 10%
Question 7.
A cubical copper block has each side 2.0 cm. It is suspended by a string and submerged in oil of density 820 kg m-3. Calculate the tension in the string.
(density of copper = 8920 kg m-3)
Answer:
Tension = true weight – upthrust due to oil
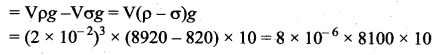
![]()
Question 8.
By sucking through a straw, a student can reduce the pressure in his lungs to 750 mm of Hg (density = 13.6 g cm-2). Using the straw, he can drink water from a glass upto a maximum depth of.
Answer:
Pressure difference between atmosphere and lungs
∆P = 760 – 750 = 10 mm = 1 cm of Hg
Maximum depth upto which the student can suck water from a glass = 1 × 13.6 = 13.6 cm
Question 9.
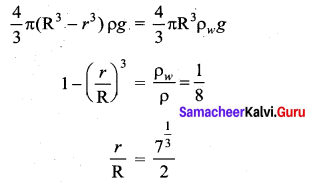
A sphere made of a material of specific gravity 8 has a concentric spherical cavity and just sinks in water. Calculate the ratio of the radius of the cavity to that of the outer radius of the sphere.
Answer:
Let the radius of the sphere be R and that of the cavity be r. Then, since the sphere just sinks in water,
Question 10.
A solid floats in liquid A with half its volume immersed and in liquid B with \(\frac{2}{3}\) of its volume immersed. The densities of the liquids A and B are in the ratio.
Answer:
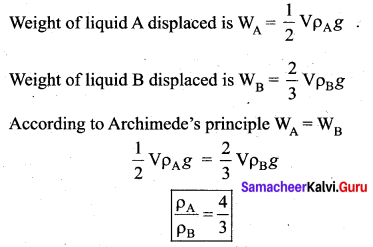
Question 11.
An ideal liquid is flowing in a cylindrical tube of internal diameter 4 cm with a velocity of 5 ms-1. If this tube is connected to another tube of internal diameter 2 cm, then the velocity of the liquid in the second tube will be?
Answer:
According to the equation of continuity
![]()
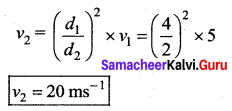
![]()
Question 12.
The cylindrical tube of a spray pump has radius R, one end of which has ‘n’ fine holes, each of radius r. If the speed of the liquid in the tube is V, what is the speed of the ejection of the liquid through the holes.
Answer:
Let the required speed be v’.
According to equation of continuity a’v’ = av
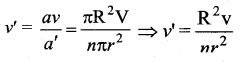
Question 13.
What is the pressure on a swimmer 10 m below the surface of a lake?
Answer:
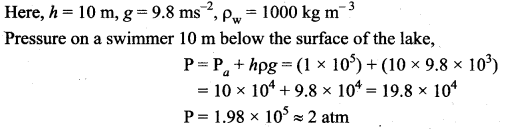
Question 14.
A square metal plate of 10 cm side moves parallel to another plate with a velocity of 10 cm s-1, both plates immersed in water. If the viscous force is 200 dyne and viscosity of water is 0.01 poise. What is their distance apart?
Answer:
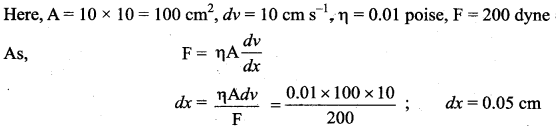
Question 15.
Find the terminal velocity of a steel ball 2 mm in diameter falling through glycerine. Relative density of steel is 8, relative density of glycerine is 1.3 and viscosity of glycerine is 8.3 poise.
Answer:
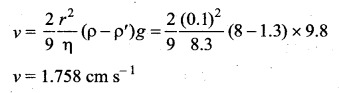
Question 16.
The terminal velocity of a copper ball of radius 2 mm falling through a tank of oil at 20°C is 6.5 cm s-1. Compute the viscosity of the oil at 20°C. Density of oil = 1.5 × 103 kg m -3, density of copper is 8.9 × 103 kg m-3.
Answer:
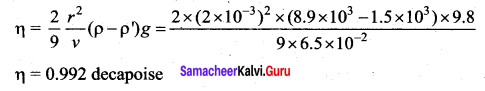
![]()
Question 17.
Water is flowing in a pipe of radius 1.5 cm with an average velocity of 15 cm s-1. What is the nature of flow? Given coefficient of viscosity of water is 10-3 kg m-1s-1 and its density
Answer:

As Rc > 3000, so the flow is turbulent.
Question 18.
Water is flowing with a speed of 2 ms-1 in a horizontal pipe with cross- sectional area decreasing from 2 × 10-2 m-2 to 0.01 m2 at pressure 4 × 104 Pa. What will be the pressure at small cross-section?
Answer:

Question 19.
Calculate the height to which water will rise in capillary tube of 1.5 mm diameter. Surface tension of water is 7.4 × 10-3 Nm-1.
Solution: