You can Download Samacheer Kalvi 11th Maths Book Solutions Guide Pdf, Tamilnadu State Board help you to revise the complete Syllabus and score more marks in your examinations.
Tamilnadu Samacheer Kalvi 11th Maths Solutions Chapter 1 Sets Ex 1.1
Question 1.
Write the following in roster form.
(i) {x ∈ N : x2 < 121 and x is a prime}.
(ii) the set of all positive roots of the equation (x – 1)(x + 1)(x2 – 1) = 0.
(iii) {x ∈ N : 4x + 9 < 52}.
(iv) {x : \(\frac{x-4}{x+2}\) = 3, x ∈ R – {-2}}
Solution:
(i) Let A = { x ∈ N : x2 < 121 and x is a prime }
A = {2, 3, 5, 7}
(ii) The set of positive roots of the equations
(x – 1) (x + 1) (x2 – 1) = 0
(x – 1 ) (x + 1 ) (x + 1) (x – 1) = 0
(x + 1 )2 (x – 1)2 = 0
(x + 1)2 = 0 or (x – 1)2 = 0
x + 1 = 0 or x – 1 = 0
x = -1 or x = 1
A = { 1 }
(iii) Let A = { x ∈ N : 4x + 9 < 52 }
When x = 1, (4) × (1 ) + 9 = 4 + 9 = 13
When x = 2, (4) × (2) + 9 = 8 + 9 = 17
When x = 3, (4) × (3) + 9 = 12 + 9 = 21
When x = 4, (4) × (4) + 9 = 16 + 9 = 25
When x = 5, (4) × (5) + 9 = 20 + 9 = 29
When x = 6, (4) × (6) + 9 = 24 + 9 = 33
When x = 7, (4) × (7) + 9 = 28 + 9 = 37
When x = 8, (4) × (8) + 9 = 32 + 9 = 41
When x = 9, (4) × (9) + 9 = 36 + 9 = 45
When x = 10, (4) × (10) + 9 = 40 + 9 = 49
∴ A = { 1, 2, 3, 4, 5, 6 ,7, 8, 9, 10 }
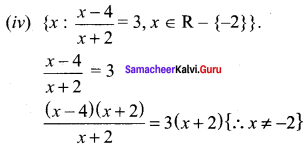
(i.e.) x – 4 = 3(x + 2)
x – 4 = 3x + 6
– 4 – 6 = 3x – x
2x = -10 ⇒ x = -5
A = {-5}
![]()
Question 2.
Write the set {-1, 1} in set builder form.
Solution:
A = {x : x2 – 1 = 0, x ∈ R}
Question 3.
State whether the following sets are finite or infinite.
- {x ∈ N : x is an even prime number}
- {x ∈ N : x is an odd prime number}
- {x ∈ Z : x is even and less than 10}
- {x ∈ R : x is a rational number}
- {x ∈ N : x is a rational number}
Solution:
- Finite set
- Infinite set
- Infinite
- Infinite
- Infinite
Question 4.
By taking suitable sets A, B, C, verify the following results:
(i) A × (B ∩ C) = (A × B) ∩ (A × C).
(if) A × (B ∪ C) = (A × B) ∪ (A × C).
(iii) (A × B) ∩ (B × A) = (A ∩ B) × (B ∩ A).
(iv) C – (B – A) = (C ∩ A) ∪ (C ∩ B).
(v) (B – A) ∩ C = (B ∩ C) – A = B ∩ (C – A).
Solution:
To prove the following results let us take U = {1, 2, 5, 7, 8, 9, 10}
A = {1, 2, 5, 7}
B = {2, 7, 8, 9}
C = {1, 5, 8, 7}
(i) Let A = {1, 2}, B = {3, 4}, C = {4, 5}
B ∩ C = {3, 4} ∩ {4, 5}
B ∩ C = {4}
A × (B ∩ C) = {1, 2} × {4}
A × (B ∩ C) = { (1,4), (2,4) } —– (1)
A × B = {1, 2} × {3, 4}
A × B = { (1,3), (1, 4), (2, 3), (2, 4)}
A × C = {1, 2} × { 4, 5 }
A × C = {(1, 4), (1, 5), (2, 4), (2, 5)}
(A × B) ∩ (A × C) = {(1, 3), (1, 4), (2, 3), (2, 4)} ∩ { (1, 4), (1, 5), (2, 4), (2, 5)}
(A × B) ∩ (A × C) = {(1, 4), (2, 4)} —- (2)
From equations (1) and (2)
A × (B ∩ C) = (A × B) ∩ (A × C)
(ii) To prove A × (B ∪ C) = (A × B) (A × C)
B = {2, 7, 8, 9}, C = {1, 5, 8, 10)
B ∪ C = {1, 2, 5, 7, 8, 9, 10}
A = {1, 2, 5, 7}
A × (B ∪ C) = {(1, 1), (1, 2), (1, 5), (1, 7), (1, 8), (1, 9), (1, 10), (2, 1), (2, 2), (2, 5), (2, 7), (2, 8), (2, 9), (2, 10), (5, 1), (5, 2), (5, 5), (5, 7), (5, 8), (5, 9), (5, 10), (7, 1), (7, 2), (7, 5), (7, 7), (7, 8), (7, 9), (7, 10)) …. (1)
A × B = {(1, 2), (1, 7), (1, 8), (1, 9), (2, 2), (2, 7), (2, 8), (2, 9), (5, 2), (5, 7), (5, 8), (5, 9),
(7, 2), (7, 7), (7, 8), (7, 9)}
A × C = {(1, 1), (1, 5), (1, 8), (1, 10), (2, 1), (2, 5), (2, 8), (2, 10), (5, 1), (5, 5), (5, 8), (5, 10), (7, 1), (7, 5), (7, 8), (7, 10)}
(A × B) ∪ (A × C) = (1, 1), (1, 2), (1, 5), (1, 7), (1, 8), (1,9), (1, 10), (2, 1), (2, 2), (2, 5), (2, 7), (2, 8), (2, 9), (2, 10), (5, 1), (5, 2), (5, 5), (5, 7), (5, 8), (5, 9), (5, 10), (7, 1), (7, 2), (7, 5), (7, 7), (7, 8), (7, 9), (7, 10)} …… (2)
(1) = (2) ⇒ A × (B ∪ C) = (A × B) ∪ (A × C)
(iii) Let A = {1, 2}, B = {2, 3}
A × B = {1, 2} × {2, 3}
A × B = {(1, 2), (1, 3), (2, 2), (2, 3)}
B × A = {2, 3} × {1, 2}
B × A = {(2, 1), (2, 2), (3, 1), (3,2)}
(A × B) ∩ (B × A) = {(1, 2), (1, 3),(2, 2), (2, 3)} ∩ {(2, 1), (2, 2), (3, 1),(3, 2)}
(A × B) ∩ (B × A) = {(2, 2)} ——- (1)
A ∩ B = {1, 2} ∩ {2, 3}
A ∩ B = {2}
B ∩ A = {2, 3} ∩ {1, 2}
B ∩ A = {2}
(A ∩ B) × (B ∩ A) = {2} × {2}
(A ∩ B) × (B ∩ A) = {(2,2)} ———- (2)
From equations (1) and (2)
(A × B) ∩ (B × A) = (A ∩ B) × (B ∩ A)
(iv) To prove C – (B – A) = (C ∩ A) ∪ (C ∩ B)
B – A = {8, 9}
C = {1, 5, 8, 10}
∴ LHS = C – (B – A) = {1, 5, 10} …… (1)
C ∩ A = {1}
U = {1, 2, 5, 7, 8, 9, 10}
B = {2, 7, 8, 9} ∴ B’ = {1, 5, 10}
C ∩ B = {1, 5, 10}
R.H.S. (C ∩ A) ∪ (C ∩ B) = {1} ∪ {1, 5, 10}
= {1, 5, 10} ……. (2)
(1) = (2) ⇒ LHS = RHS
(v) Let A = {1, 2, 3, 4} , B = {3, 4, 5, 6}, C = { 5, 6, 7, 8 )
B – A = {3, 4, 5, 6} – {1, 2, 3, 4}
B – A = {5, 6}
(B – A) ∩ C = {5, 6} ∩ {5, 6, 7, 8}
(B – A) ∩ C = {5, 6} ——– (1)
(B ∩ C) = {3, 4, 5, 6} ∩ {5, 6, 7, 8}
B ∩ C = {5, 6}
(B ∩ C) – A = {5, 6} – {1,2,3,4}
(B ∩ C) – A = {5, 6} ——- (2)
C – A = {5, 6, 7, 8} – {1, 2, 3, 4}
C – A = {5, 6, 7, 8}
B ∩ (C – A) = {3, 4, 5, 6} ∩ {5, 6, 7, 8}
B ∩ (C – A) = {5, 6} ——– (3)
From equations (1) , (2) and (3)
(B – A) ∩ C = (B ∩ C) – A = B ∩(C – A)
(vi) To prove (B – A) ∪ C ={1, 5, 8, 9, 10}
B – A = {8, 9},
C = {1, 5, 8, 10}
(B – A) ∪ C = {1, 5, 8, 9, 10} ……. (1)
B ∪ C = {1, 2, 5, 7, 8, 9, 10}
A – C = {2, 7}
(B ∪ C) – (A – C) = {1, 5, 8, 9, 10} ……… (2)
(1) = (2)
⇒ (B – A) ∪ C = (B ∪ C) – (A – C)
Question 5.
Justify the trueness of the statement.
“An element of a set can never be a subset of itself.”
Solution:
“An element of a set can never be a subset of itself ”
The statement is correct
Let A = {a, b, c, d} for a ∈ A
‘a’ cannot be a subset of ‘a’
Question 6.
If n(P(A)) = 1024, n(A ∪ B) = 15 and n(P(B)) = 32, then find n(A ∩ B).
Solution:
n(P( A)) = 1024 = 210 ⇒ n( A) = 10
n(A ∪ B) = 15
n(P(B)) = 32 = 25 ⇒ n(B) = 5
We know n(A ∪ B) = n{A) + n(B) – n(A ∩ B)
(i.e.) 15 = 10 + 5 – n(A ∩ B)
⇒ n(A ∩ B) = 15 – 15 = 0
Question 7.
If n(A ∩ B) = 3 and n(A ∪ B) = 10, then find n(P(A(A ∆ B)).
Solution:
Given n(A ∩ B) = 3 and n(A ∪ B) = 10
A ∆ B = (A – B) ∪ (B – A)
n(A ∆ B) = n [ (A – B ) ∪ (B – A)]
n(A ∆ B) = n(A – B) + n(B – A) —— (1)
(Since A – B and B – A are disjoint sets)
A ∪ B = (A – B) ∪ (B – A) ∪ (A ∩ B)
n(A ∪ B) = n[(A – B) ∪ (B – A) ∪ (A ∩ B)]
n(A ∪ B) = n (A – B) + n (B – A) + n (A ∩ B)
(Since A – B, B – A and A ∩ B are disjoint sets)
n(A ∪ B) = n(A ∆ B) + n(A ∩ B)
10 = n(A ∆ B) + 3
n(A ∆ B) = 10 – 3 = 7
∴ n(P(A ∆ B)) = 27 = 128
![]()
Question 8.
For a set A, A × A contains 16 elements and two of its elements are (1, 3) and (0, 2). Find the elements of A.
Solution:
A × A = 16 elements = 4 × 4
⇒ A has 4 elements
∴ A = {0, 1, 2, 3}
Question 9.
Let A and B be two sets such that n(A) = 3 and n(B) = 2. If (x, 1), (y, 2), (z, 1) are in A × B, find A and B, where x, y, z are distinct elements.
Solution:
Given A and B be two sets such that n (A) = 3 and n(B) = 2.
Also given (x, 1), (y, 2), (z, 1) ∈ A × B
A = { x, y, z }, B = {1, 2}
Question 10.
If A × A has 16 elements, S = {(a, b) ∈ A × A : a < b} ; (-1, 2) and (0, 1) are two elements of S, then find the remaining elements of S.
Solution:
n(A × A) = 16 ⇒ n( A) = 4
S ={(-1, 0), (-1, 1), (0, 2), (1, 2)}
Samacheer Kalvi 11th Maths Solutions Chapter 1 Sets Ex 1.1 Additional Questions
Question 1.
Write the following sets in roster form
(a) {x ∈ N; x3 < 1000}
(b) {The set of positive roots of the equation (x2 – 4) (x3 – 27) = 0}
Solution:
(a) A = {1, 2, 3, 4, 5, 6, 7, 8, 9}
(b) B = {2, 3}
![]()
Question 2.
By taking suitable sets A, B, C verify the following results
(i) A × (B ∪ C) = (A × B) ∪ (A × C)
(ii) (B – A) ∪ C = (B ∪ C) – (A – C)
Solution:
Prove by yourself
Question 3.
Given n(A) = 7; n(B) = 8 and n(A ∪ B) = 10 find n[P(A ∩ B)].
Solution:
n(A ∪ B) = n(A) + n(B) – n(A ∩ B)
(i.e.,) 10 = 7 + 8 – n(A ∩ B)
⇒ n(A ∩ B) = 7 + 8 – 10 = 5
So n[P(A ∩ B)] = 25 = 32