You can Download Samacheer Kalvi 12th Maths Book Solutions Guide Pdf, Tamilnadu State Board help you to revise the complete Syllabus and score more marks in your examinations.
Tamilnadu Samacheer Kalvi 12th Maths Solutions Chapter 3 Theory of Equations Ex 3.6
Question 1.
Discuss the maximum possible number of positive and negative roots of the polynomial equation 9x9 – 4x8 + 4x7 – 3x6 + 2x5 + x3 + 7x2 + 7x + 2 = 0.
Solution:
Clearly, there are 4 sign changes for the given polynomial P(x) and hence a number of positive roots of P(x) can’t be more than four.
P(x) = 9x9 – 4x8 + 4x7 – 3x6 + 2x5 + x³ + 7x² + 7x + 2 = 0
There are two sign changes. Hence the number of negative roots can’t be more than two.
∴ It has atmost 4 positive roots and atmost two negative roots.
Question 2.
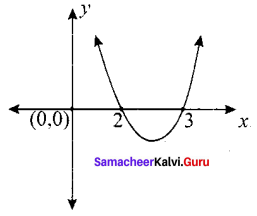
Discuss the maximum possible number of positive and negative zeros of the polynomials x2 – 5x + 6 and x2 – 5x + 16. Also, draw a rough sketch of the graphs.
Solution:
P(x) = x2 – 5x + 6
The number of sign changes in P(x) is 2.
P(x) has atmost 2 positive roots. P(-x) = x2 + 5x + 6.
The number of sign changes in P(-x) is 0.
∴ P (x) has no negative roots. P (x) = x2 – 5x + 16
![]()
Question 3.
Show that the equation x9 – 5x5 + 4x4 + 2x2 +1 = 0 has atleast 6 imaginary solutions.
Solution:
P(x) has only one sign change. It has atmost one positive roots.
P(-x) = -x9 + 5x5 + 4x4 + 2x² + 1 = 0
It has only one sign change.
It has atmost one negative roots.
Clearly 0 is not a root.
So maximum number of real roots is 3 and hence there are atleast six imaginary solutions.
Question 4.
Determine the number of positive and negative roots of the equation x9 – 5x8 – 14x7 = 0.
Solution:
x9 – 5x8 – 14x7 = 0
P (x) = x9 – 5x8 – 14x7. The number of sign changes is P(x) is 1.
The number of positive roots is 1. P (-x) = -x9 – 5x8 + 14x7
The number of sign changes is P(-x) is one. The number of negative zero of P(-x) is 1. It is clear that 0 is a root of the equation.
∴ The number of the imaginary roots is at least 6.
Question 5.
Find the exact number of real zeros and imaginary of the polynomial x9 + 9x7 + 7x5 + 5x3 + 3x.
Solution:P(x) = x9 + 9x7 + 7x5 + 5x³ + 3x = 0
P(-x) = -x9 – 9x7 – 7x5 – 5x³ – 3x
It has no sign changes.
So it has no negative real roots.
It has no positive real roots and no negative real roots.
Samacheer Kalvi 12th Maths Solutions Chapter 3 Theory of Equations Ex 3.6 Additional Problems
Question 1.
Find the maximum possible number of real roots of the equation, x5 – 6.x2 – 4x + 5 = 0.
Solution:
Let f(x) = x5 – 6x2 – 4x + 5
Check the terms when it changes sign
Number of changes = 2
∴ Maximum number of positive real roots = 2
f(-x) = (- x)5 – 6 (- x)2 – 4 (-x) + 5
= -x5 – 6x2 + 4x + 5
Check the terms when it changes signs.
Number of changes = 1
∴ Maximum number of negative real roots = 1
∴ Total maximum number of real roots = 2 + 1 = 3
Question 2.
Find the number of real roots of the equation, x2 + 5 |x| + 6 = 0.
Solution:
|x2| – 5|x| + 6 = 0
(|x| -2) (|x| -3) = 0

It has four real roots. The real roots are 2, -2, 3, -3
![]()
Question 3.
Find the real roots of the equation, x2 + 5 |x| + 6 = 0.
Solution:
x2 + 5 |x| + 6 = 0
Case (i) If x ≥ 0
x2 + 5x + 6 = 0
(x + 2) (x + 3) = 0
x = -2 and x = -3
Case (ii) If x < 0
x2 – 5x + 6 = 0
(x – 2) (x – 3) = 0
x = 2 and x = 3
Question 4.
Solve x4 – 4x2 + 8x + 35 = 0. Given (2 + \(i \sqrt{3}\)) is a root.
Solution:
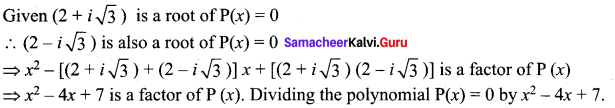
⇒ x2 – 4x + 7 is a factor of P (x). Dividing the polynomial P(x) = 0 by x2 – 4x + 7.
We get x2 + 4x + 5 = 0 is a other factor. The roots of x2 + 4x + 5 = 0 are
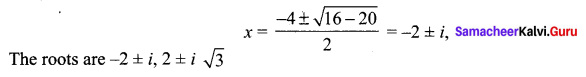
Question 5.
Solve x4 – 5x3 + 4x2 + 8x – 8 = 0. Given (1 – \(\sqrt{5}\)) is a root of the polynomial equation.
Solution:
Since (1 – \(\sqrt{5}\)) is a root of the polynomial P(x) = 0
(1 + \(\sqrt{5}\)) is also a root of P (x) = 0
⇒ x2 – [(1 + \(\sqrt{5}\)) + (1 – \(\sqrt{5}\))] x + (1 + \(\sqrt{5}\) ) (1 – \(\sqrt{5}\) ) = 0 is a factor of P(x) = 0 ⇒ x2 – 2x – 4 = 0 is a factor of P(x) = 0.
Dividing the polynomial by x2 – 2x – 4 = 0
We get the other factor x2 – 3x + 2 = 0
The roots of x2 – 3x + 2 = 0
(x – 2) (x – 1) = 0
x = 1, 2
The roots are 1, 2, 1 ± \(\sqrt{5}\)
![]()
Question 6.
Find a polynomial equation of the lowest degree with rational co-efficient having \(\sqrt{3}\), (1 – 2i) as two of its roots.
Solution:
When \(\sqrt{3}\) is a root, – \(\sqrt{3}\) will also be a root.
Now the quadratic equation with \(\sqrt{3}\) , – \(\sqrt{3}\) are roots is x2 – (\(\sqrt{3}\) – \(\sqrt{3}\))x + (\(\sqrt{3}\))(-\(\sqrt{3}\)) = 0
(i.e) x2 – 3 = 0
When 1 – 2i is a root, 1 + 2i will be another root.
Now the quadratic equation with roots 1 – 2i and 1 + 2i is
x2 – (1 – 2i + 1 + 2i)x + (1 – 2i)(1 + 2i) = 0
(i.e) x2 – 2x + 5 = 0
∴ The equation with roots ± \(\sqrt{3}\) and 1 ± 2i is (x2 – 3) (x2 – 2x + 5) = 0
(i.e) x4 – 2x3 + 5x2 – 3x2 + 6x – 15 = 0 ,
(i.e) x4 – 2x3 + 2x2 + 6x – 15 = 0