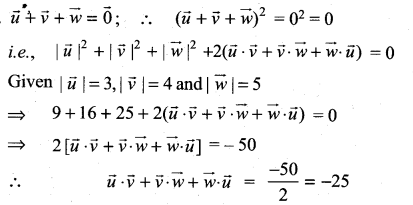You can Download Samacheer Kalvi 11th Maths Book Solutions Guide Pdf, Tamilnadu State Board help you to revise the complete Syllabus and score more marks in your examinations.
Tamilnadu Samacheer Kalvi 11th Maths Solutions Chapter 8 Vector Algebra – I Ex 8.3
Question 1.
Find \(\vec{a} \cdot \vec{b}\) when
![]()
Solution:
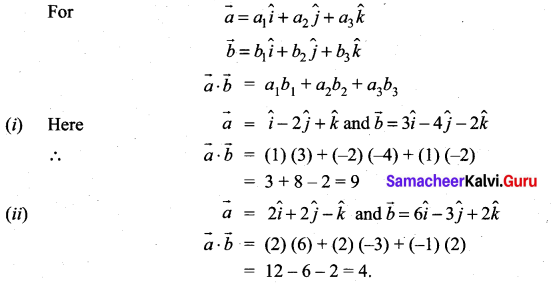
Question 2.
Find the value of λ for which the vectors \(\vec{a}\) and \(\vec{b}\) are perpendicular, where
![]()
Solution:
(i) \(\vec{a}\) = 2î + λĵ – k̂ and \(\vec{b}\) = î – 2ĵ + 3k̂
Given \(\vec{a}\) and \(\vec{b}\) are perpendicular vectors
∴ \(\vec{a}\) . \(\vec{b}\) = 0
(2î + λĵ – k̂ ) . (î – 2ĵ + 3k̂) = 0
(2) (1) + (λ) (- 2) + (1) (3) = 0
2 – 2λ + 3 = 0
2λ = 5
λ = \(\frac{5}{2}\)
(ii) \(\vec{a}\) = 2î + 4ĵ – k̂ and \(\vec{b}\) = 3î – 2ĵ + λk̂
Given \(\vec{a}\) and \(\vec{b}\) are perpendicular vectors
∴ \(\vec{a}\) . \(\vec{b}\) = 0
(2î + 4ĵ – k̂) . (3î – 2ĵ + λk̂) = 0
(2) (3) + (4) (-2) + (-1) (λ) = 0
6 – 8 – λ = 0
λ = – 2
Question 3.
If \(\vec{a}\) and \(\vec{b}\) are two vectors such that |\(\vec{a}\)| = 10, |\(\vec{b}\)| = 15 and \(\vec{a} \cdot \vec{b}\) = 75\(\sqrt{2}\) , find the angle between \(\vec{a}\) and \(\vec{a}\) .
Solution:
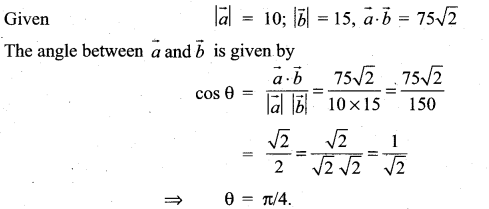
![]()
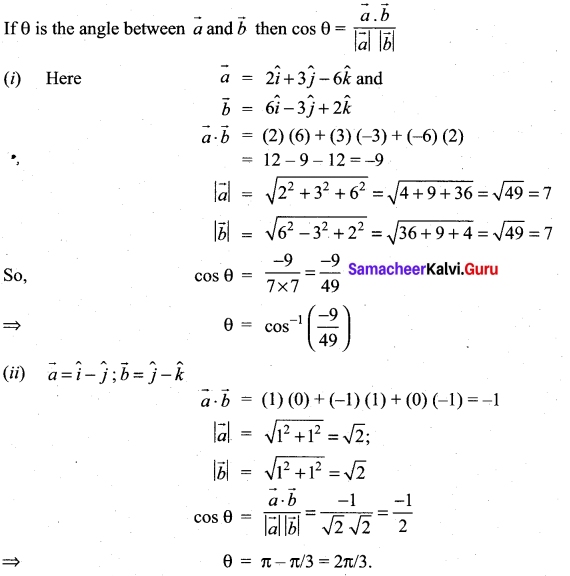
Question 4.
Find the angle between the vectors
![]()
Solution:
Question 5.
If \(\overrightarrow{\boldsymbol{a}}, \overrightarrow{\boldsymbol{b}}, \overrightarrow{\boldsymbol{c}}\) are three vectors such that \(\vec{a}+2 \vec{b}+\vec{c}=\overrightarrow{0}\) and \(|\vec{a}|=3,|\vec{b}|=4,|\vec{c}|=7\) find the angle between \(\vec{a}\) and \(\vec{b}\)
Solution:

Question 6.
Show that the vectors ![]() are mutually orthogonal.
are mutually orthogonal.
Solution:

Question 7.
Show that the vectors ![]() form a right-angled triangle.
form a right-angled triangle.
Solution:
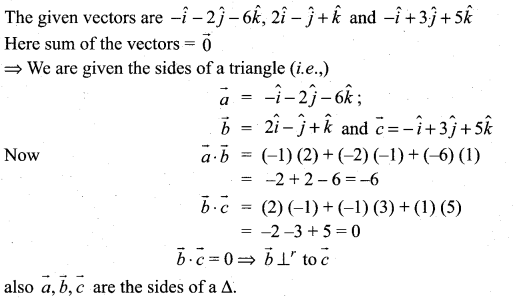
So, the given vectors form the sides of a right-angled triangle
Question 8.
![]()
Solution:
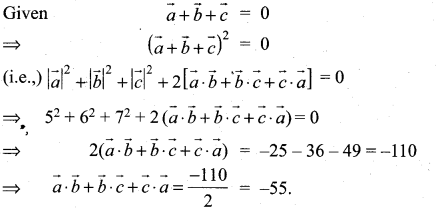
Question 9.
Show that the points (2, -1, 3) (4, 3, 1) and (3, 1, 2) are collinear
Solution:
Let the given points be A, B, C
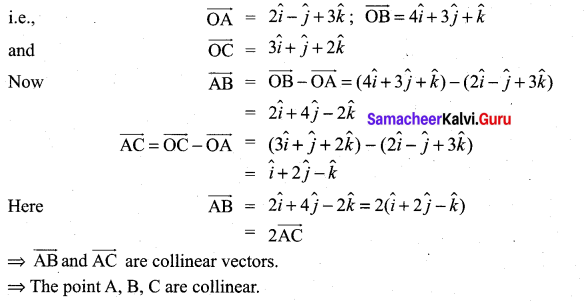
![]()
Question 10.
If \(\vec{a}, \vec{b}\) are unit vectors and θ is the angle between them, show that
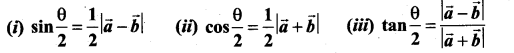
Solution:
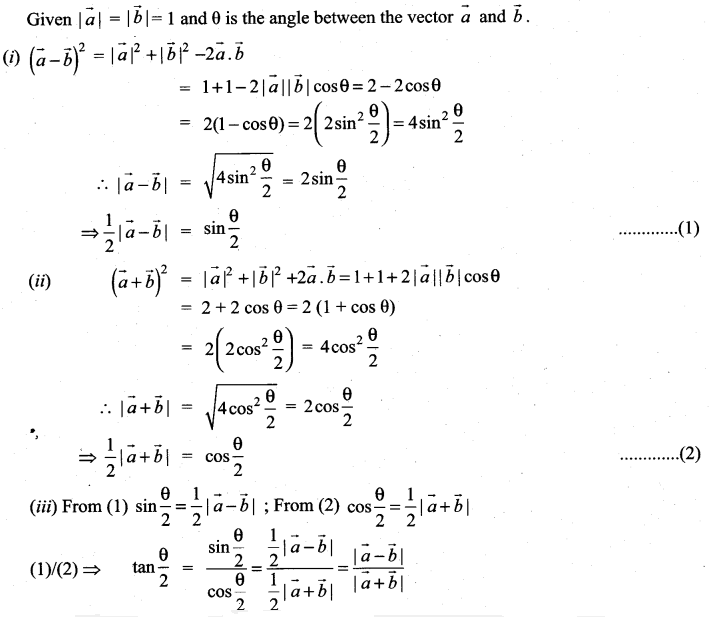
Question 11.
Let \(\vec{a}, \vec{b}, \vec{c}\) be the three vectors such that \(|\vec{a}|=3,|\vec{b}|=4,|\vec{c}|=5\) and each one of them being perpendicular to the sum of the other two, find \(|\vec{a}+\vec{b}+\vec{c}|\)
Solution:
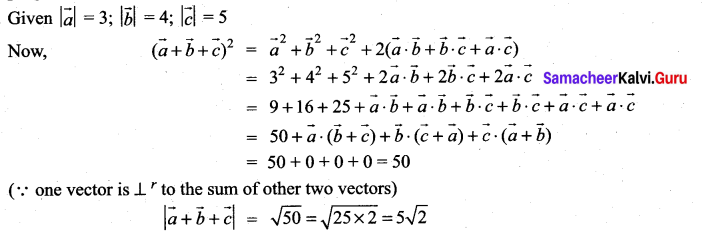
Question 12.
Find the projection of the vector \(\hat{i}+3 \hat{j}+7 \hat{k}\) on the vector \(2 \hat{i}+6 \hat{j}+3 \hat{k}\)
Solution:
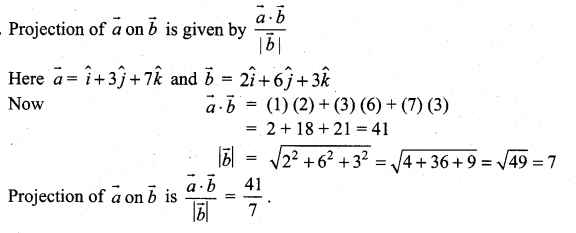
Question 13.
Find λ, when the projection of ![]() is 5 units.
is 5 units.
Solution:

Question 14.
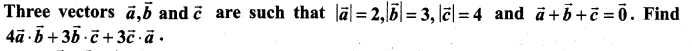
Solution:
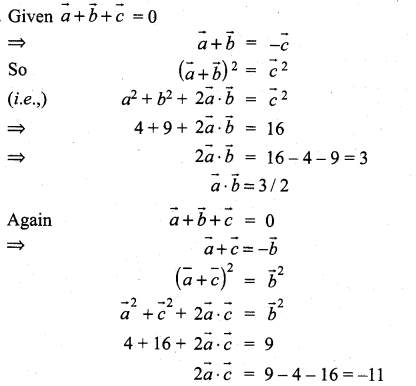
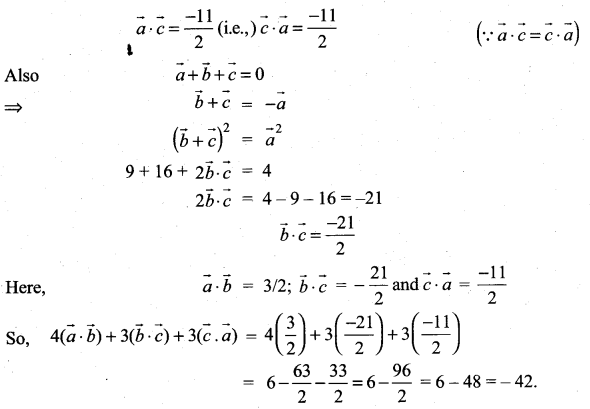
Samacheer Kalvi 11th Maths Solutions Chapter 8 Vector Algebra – I Ex 8.3 Additional Problems
Question 1.
Find λ so that the vectors ![]() are perpendicular to each other.
are perpendicular to each other.
Solution:
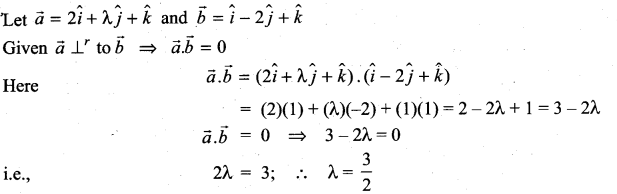
![]()
Question 2.
![]()
Solution:
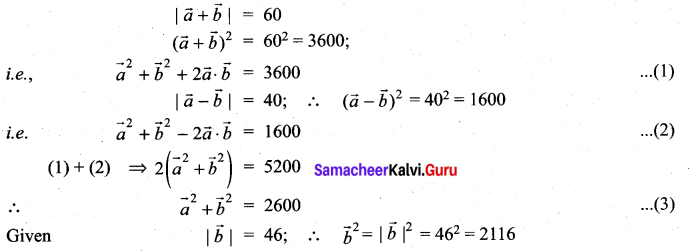
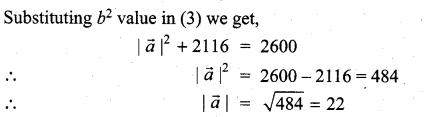
Question 3.
If the sum of two unit vectors is a unit vector prove that the magnitude of their difference is \(\sqrt{3}\)
Solution:

Question 4.
Show that the vectors ![]() form a right-angled triangle.
form a right-angled triangle.
Solution:
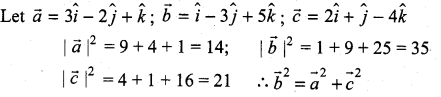
⇒ The given vectors form the sides of a right-angled triangle.
![]()
Question 5.
Find the projection of
![]()
Solution:
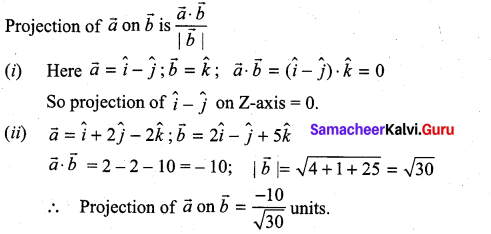
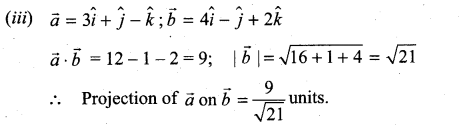
Question 6.
Show that the vector \(\hat{i}+\hat{j}+\hat{k}\) is equally inclined with the coordinate axes.
Solution:
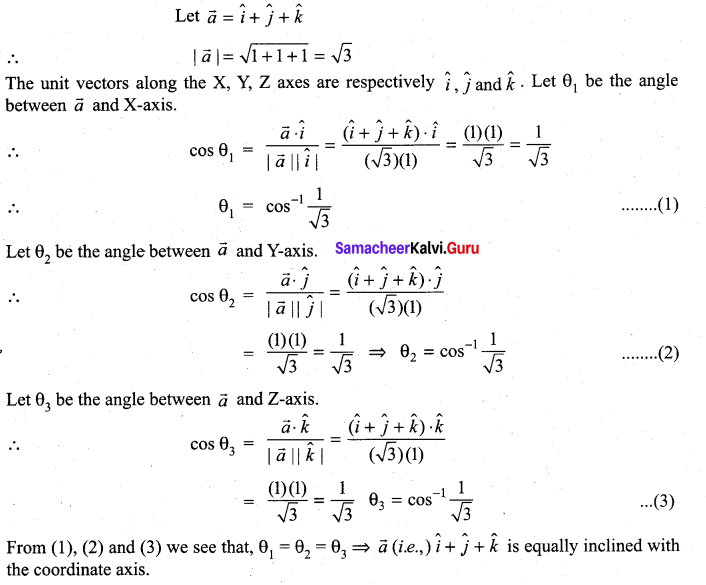
Question 7.
If \(\vec{a}, \vec{b}, \vec{c}\) are three mutually perpendicular unit vectors, then prove that \(|\vec{a}+\vec{b}+\vec{c}|=\sqrt{3}\)
Solution:
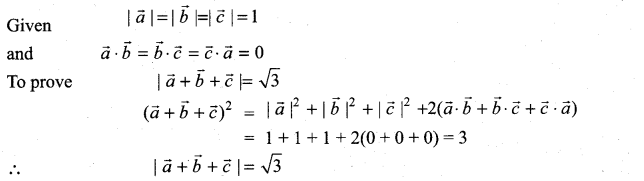
Question 8.
Show that the points whose positions vectors ![]() from a right-angled triangle.
from a right-angled triangle.
Solution:
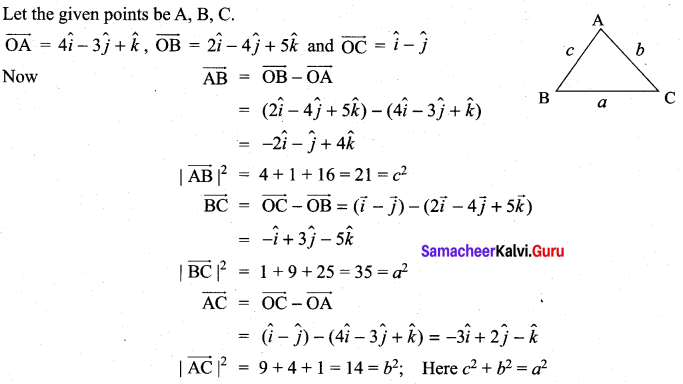
⇒ The given points form a right-angled triangle.
Question 9.
![]()
Solution: