Samacheer Kalvi 12th Maths Solutions Chapter 5 Two Dimensional Analytical Geometry – II Ex 5.4

You can Download Samacheer Kalvi 12th Maths Book Solutions Guide Pdf, Tamilnadu State Board help you to revise the complete Syllabus and score more marks in your examinations.

Tamilnadu Samacheer Kalvi 12th Maths Solutions Chapter 5 Two Dimensional Analytical Geometry – II Ex 5.4

Question 1.
Find the equations of the two tangents that can be drawn from (5, 2) to the ellipse 2x² + 7y² = 14 .
Solution:
2x² + 7y² = 14
\(\frac {x^2}{7}\) + \(\frac {y^2}{2}\) = 1
y = mx + \(\sqrt { a^2m^2+b^2 }\)
a² = 7, b² = 2
It passes through (5, 2)
2 = 5 m + \(\sqrt { 7m^2 + 2 }\)
2 – 5 m = \(\sqrt { 7m^2 + 2 }\)
(2 – 5 m)² = (7m² + 2)
4 + 25m² – 20m = 7m² + 2
18m² – 20m + 2 = 0
9m² – 10m + 1 = 0
(9m – 1) (m – 1) = 0
m = 1 (or) m = \(\frac {1}{9}\)
Equation of tangent
(i) m= 1 ⇒ (y – 2) = 1(x- 5)
x – y – 3 = 0
(ii) m = \(\frac {1}{9}\) ⇒ y – 2 = \(\frac {1}{9}\) (x – 5)
9y – 18 = x – 5
x – 9y + 13 = 0
Equation of tangent be x – y – 3 = 0 and x – 9y + 13 = 0.

Question 2.
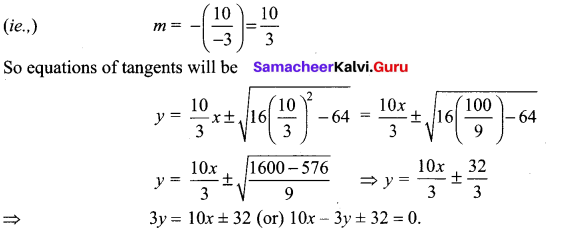
Find the equations of tangents to the hyperbola \(\frac{x^{2}}{16}-\frac{y^{2}}{64}\) = 1 which are parallel to 10x – 3y + 9 = 0.
Solution:
\(\frac{x^{2}}{16}-\frac{y^{2}}{64}\) = 1
Here a² = 16 and b² = 64
The equation of tangents will be of the form y = mx ± \(\sqrt{a^{2} m^{2}-b^{2}}\)
(i.e.,) y = mx ± \(\sqrt{16 m^{2}-64}\)
Where ‘m’ is the slope of the tangent.
Here we are given that the tangents are parallel to 10x – 3y + 9 = 0
So the slope of tangents will be equal to the slope of the given line

Question 3.
Show that the line x – y + 4 = 0 is a tangent to the ellipse x² + 3y² = 12. Also find the coordinates of the point of contact.
Solution:
Equation of the ellipse
x² + 3y² = 12
÷ by 12 ⇒ \(\frac {x^2}{12}\) + \(\frac {y^2}{4}\) = 1
a² = 12 b² = 4
Equation of the line
x – y + 4 = 0
y = x + 4
m = 1, c = 4
Condition that the line y = mx + c to be tangent to the ellipse is
c² = a²m² + b²
4² = 12(1)² + 4
16 = 16
∴ The line is a tangent to the ellipse.
Point of contact = (\(\frac {-a^2m}{c}\), \(\frac {b^2}{c}\))
= (\(\frac {-12(1)}{4}\), \(\frac {4}{4}\))
= (-3, 1)

Question 4.
Find the equation of the tangent to the parabola y² = 16x perpendicular to 2x + 2y + 3 =0
Solution:
y² =16x
Comparing this equation with y² = 4ax
we get 4a = 16 ⇒ a = 4
The equation of tangent to the parabola y² = 16x will be of the form

So m = 1
⇒ The equation of tangent will be y = 1(x) + \(\frac{4}{1}\) (i.e.,) y = x + 4
(or) x – y + 4 = 0

Question 5.
Find the equation of the tangent at t = 2 to the parabola y² = 8x. (Hint: use parametric form)
Solution:
Equation of a tangent be
yt = x + at² Here t = 2
y² = 8x ∴ 4a = 8
a = 2
y(2) = x + 2(4)
2y = x + 8
x – 2y + 8 = 0

Question 6.
Find the equations of the tangent and normal to hyperbola 12x² – 9y² = 108 at θ = \(\frac{\pi}{3}\) .
(Hint: Use parametric form)
Solution:
12x² – 9y² = 108

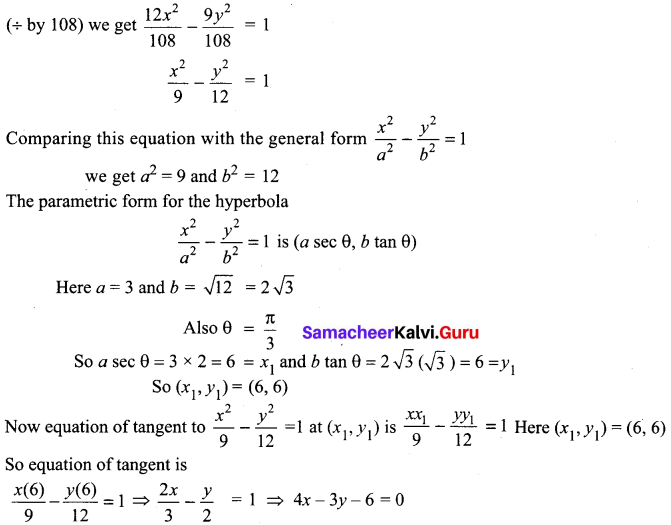
Normal is a line perpendicular to tangent
So equation of normal will be of the form 3x + 4y + k = 0
The normal is drawn at (6, 6)
⇒ 18 + 24 + k = 0 ⇒ k = – 42
So equation of normal is 3x + 4y – 42 = 0

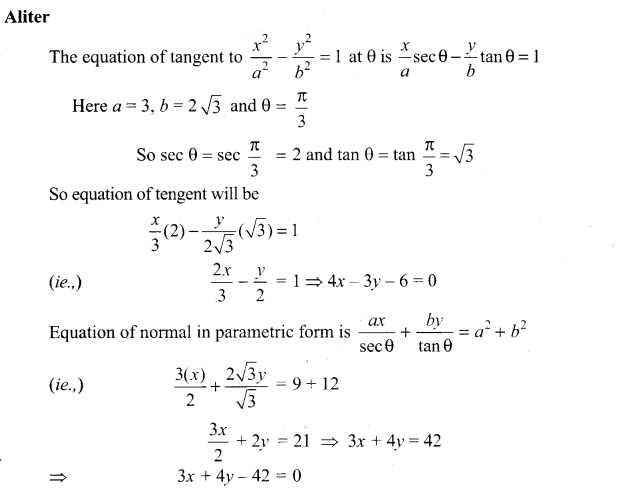
Question 7.
Prove that the point of intersection of the tangents at ‘t₁‘ and t₂’ on the parabola y² = 4ax is [at₁ t₂, a (t₁ + t₂)].
Solution:
The equation of tangent to y² = 4ax at ‘t’ is given by yt = x + at²
So equation of tangent at ‘t₁‘ is yt₁ = x + at₁²
and equation of tangent at ‘t₂‘ is yt₂ = x + at₂²
To find the point of intersection we have to solve the two equations

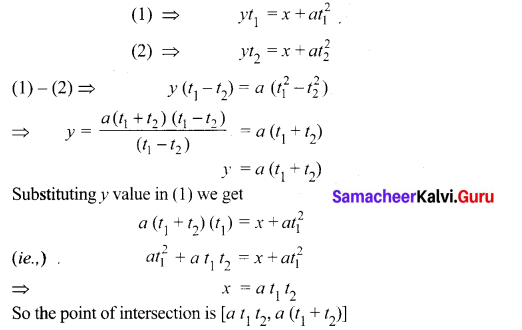
Question 8.
If the normal at the point ‘t₁‘ on the parabola y² = 4ax meets the parabola again at the point ‘t₂‘, then prove that t₂ = \(-\left(t_{1}+\frac{2}{t_{1}}\right)\)
Solution:
Normal equation of the parabola y² = 4ax at t₁ be
y + xt₁ = at₁³ + 2at₁
It meets the point t₂ {at₂², 2at₂)
2 at₂ + at₂²t₁ = at₁³ + 2 at₁
at₂²t₁ – at₁³ = 2at₁ – 2at₂
at₁(t₂² – t₁²) = -2a(t₂ – t₁)
at₁(t₂ + t₁)(t₂ – t₁) = -2a(t₂ – t₁)
t₂ + t₁ = \(\frac {-2}{t_1}\)
t₂ = \(\frac {-2}{t_1}\) – t₁
t₂ = -(\(\frac {2}{t_1}\) + t₁)

Samacheer Kalvi 12th Maths Solutions Chapter 5 Two Dimensional Analytical Geometry – II Ex 5.4 Additional Questions

Question 1.
Find the equations of the tangent and normal to the parabolas: x² + 2x – 4y + 4 = 0 at (0, 1)
Solution:

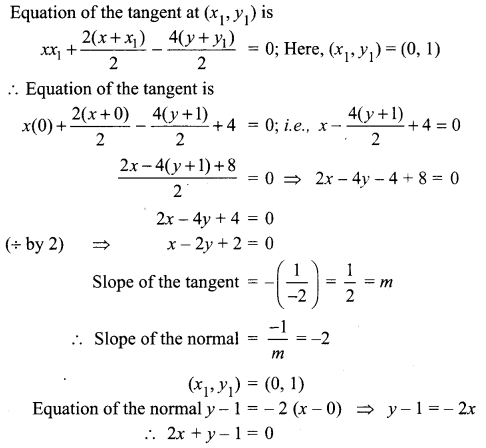
Question 2.
Find the equations of the tangent and normal to the parabola y² = 8x at t = \(\frac{1}{2}\)
Solution:


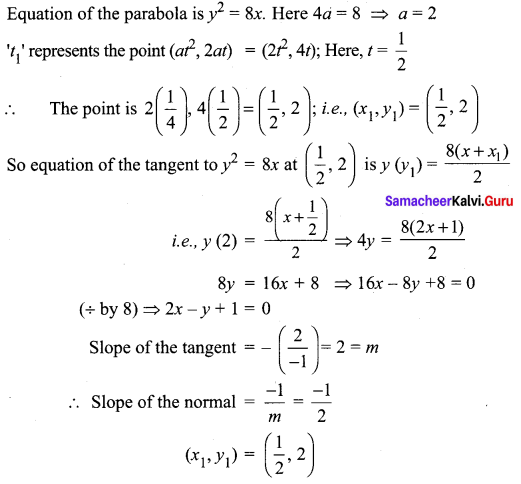
∴ Equation of the tangent is 2x – y + 1 = 0 and equation of the normal is 2x + 4y – 9 = 0

Question 3.
Find the equations of the tangents: to the parabola y² = 6x, parallel to 3x – 2y + 5 = 0
Solution:


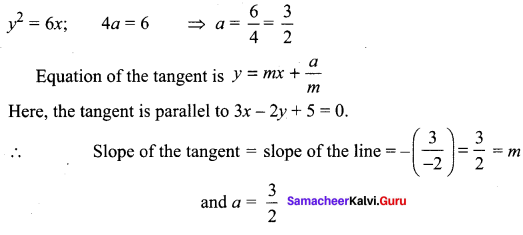
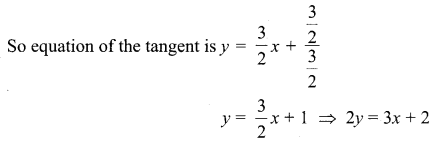
∴ Equation of the tangent is 3x – 2y + 2 = 0

Question 4.
Find the equations of the tangents: to the parabola 4x² – y² = 64 Which are parallel to 10x – 3y + 9 = 0.
Solution:

Question 5.
Find the equation of the tangent from point (2, -3) to the parabola y² = 4x.
Solution:
y² = 4x
Equation of the tangent to the parabola will be of the form y = \(m x+\frac{1}{m}\)
The tangents pass through (2, -3)


The two tangents drawn from (2, -3) are x + y + 1 = 0, x + 2y + 4 = 0

Question 6.
Find the equation of the tangents from the point (2, -3) to the parabola 2x² – 3y² = 6
Solution:
2x² – 3y² = 6

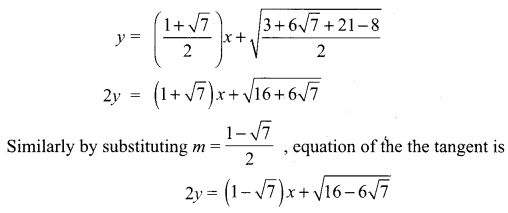
Question 7.
Prove that the line 5x + 12y = 9 touches the hyperbola x² – 9y² = 9 and find the point of contact.
Solution:

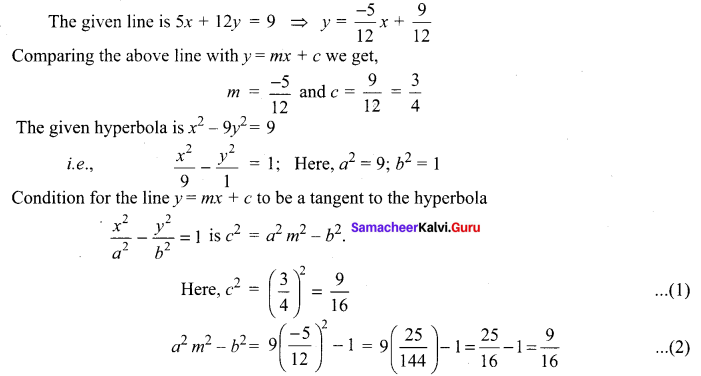
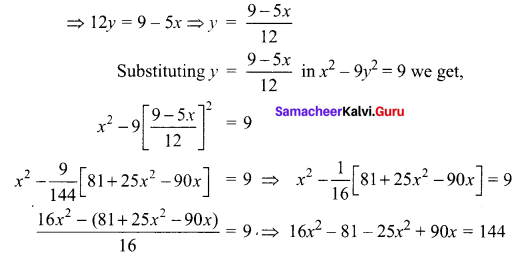
(1) = (2) ⇒ The given line is a tangent to the curve i.e., the given line touches the curve. To find the point of contact we have to solve the line and the curve.
The given line 5x + 12y = 9

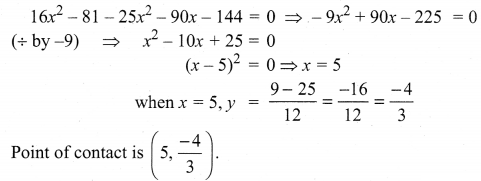
Question 8.
Show that the line x – y + 4 = 0 is a tangent to the ellipse x² + 3y² = 12. Find the co-ordinates of the point of contact.
Solution:
The given ellipse is x² + 3y² = 12
Given line is x – y + 4 = 0 ⇒ y = x + 4
Here, m = 1 and c = 4
The condition for the line y = mx + c to be a tangent to the ellipse

RHS: a² m² + b = 12(1) + 4 = 16
LHS: RHS ⇒ the given lines is a tangent to the ellipse.