You can Download Samacheer Kalvi 11th Maths Book Solutions Guide Pdf, Tamilnadu State Board help you to revise the complete Syllabus and score more marks in your examinations.
Tamilnadu Samacheer Kalvi 11th Maths Solutions Chapter 2 Basic Algebra Ex 2.3
Question 1.
Represent the following inequalities in the interval notation:
Solution:
![]()
⇒ x ∈ [-1, 4)
[] closed interval, end points are included
() ➝ open interval
end points are excluded
(ii) x ≤ 5 and x ≥ -3[i] x ≤ 5 and x ≥ -3
Solution:

x ∈ [-3, 5)
(iii) x < -1 or x < 3
Solution:
![]()
x ∈ (-∞, -1) or x ∈ (-∞, 3)
(iv) – 2x > 0 or 3x – 4 < 11
Solution:
-2x > 0 ⇒ 2x < 0 ⇒ x < 0
x ∈ (-∞, 0)
3x – 4 < 11
⇒ 3x – 4 + 4 < 11 + 4
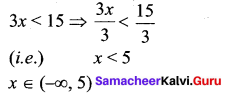
Question 2.
Solve 23x < 100 when
(i) x is a natural number,
(ii) x is an integer.
Solution:
23x <100
![]()
(i.e.,) x > 4.3
(i) x = 1, 2, 3, 4 (x ∈ N)
(ii) x = …. -3, -2, -1, 0, 1, 2, 3, 4 (x ∈ Z)
Question 3.
Solve -2x ≥ 9 when
(i) x is a real number,
(ii) x is an integer,
(iii) x is a natural number.
Solution:
-2x > 9 ⇒ 2x ≤ -9

(ii) x = …. -3, -2, -1, 0, 1, 2, 3, 4
(iii) x = 1, 2, 3, 4
Question 4.
![]()
Solution:
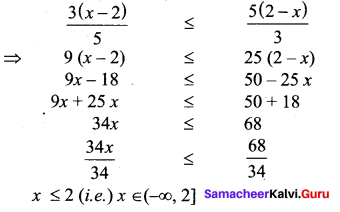
(ii)
![]()
Solution:
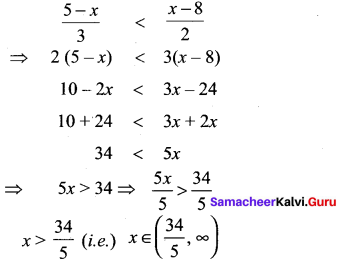
![]()
Question 5.
To secure an A grade one must obtain an average of 90 marks or more in 5 subjects each of a maximum of 100 marks. If one scored 84, 87, 95, 91 in the first four subjects, what is the minimum mark one scored in the fifth subject to get an A grade in the course?
Solution:
Given, to secure A grade in 5 subjects required average mark of 90 or more.
The marks scored in the first four subjects are 84, 87, 95, 91
Let the marks scored in the fifth subject be.
Then by the given data, we have
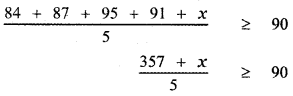
Multiplying both sides by 5, we get
357 + x ≥ 450
x ≥ 450 – 357
x ≥ 93
∴ The person must obtain a minimum of 93 marks to get A grade in the course.
Question 6.
A manufacturer has 600 litres of a 12 percent solution of acid. How many litres of a 30 percent acid solution must be added to it so that the acid content in the resulting mixture will be more than 15 percent but less than 18 percent?
Solution:
12% solution of acid in 600 l ⇒ 600 × \(\frac{12}{100}\) = 72 l of acid
15% of 600 l ⇒ 600 × \(\frac{15}{100}\) = 90 l
18% of 600 l ⇒ 600 × \(\frac{18}{100}\) = 108 l
Let x litres of 18% acid solution be added

(600 + x)15 ≥ 7200 + 30x
9000+ 15x ≥ 7200 + 30x
1800 ≥ 15x
x ≤ 120
Let x litres of 18% acid solution be added
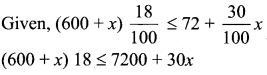
10800 + 18 ≤ 7200 + 30x
3600 ≤ 12x
x > 300
The solution is 120 ≤ x > 300
![]()
Question 7.
Find all pairs of consecutive odd natural numbers both of which are larger than 10 and their sum is less than 40.
Solution:
Let the two numbers be x and x + 2
x + x + 2 < 40
⇒ 2x < 38

⇒ x< 19 and x > 10
so x = 11 ⇒ x + 2 = 13
x = 13 ⇒ x + 2 = 15
x = 15 ⇒ x + 2 = 17
When x = 17 ⇒ x + 2 = 19
So the possible pairs are (11, 13), (13, 15), (15, 17), (17, 19)
Question 8.
A model rocket is launched from the ground. The height h of the rocket after t seconds from lift off is given by h(t) = -5t2 + 100t; 0 ≤ r ≤ 20. At what time the rocket is 495 feet above the ground?
Solution:
Given h(t) = – 5t2 + 100t, 0 ≤ t ≤ 20.
Let the time be ‘t’ sec when the rocket is 495 feet above the ground.
∴ h (t) = 495 for time ‘t’ sec
-5t2 + 100t = 495
5t2 – 100t + 495 = 0
t2 – 20t + 99 = 0
t2 – 11t – 9t + 99 = 0
t(t – 11) – 9(t – 11) = 0
(t – 9 ) (t – 11 ) = 0
t – 9 = 0 or t – 11 = 0
t = 9 or t = 11
∴ At t = 11 or 9 seconds, the rocket is 495 feet above the ground.
![]()
Question 9.
A Plumber can be paid according to the following schemes: In the first scheme he will be paid Rs. 500 plus Rs.70 per hour, and in the second scheme he will be paid Rs. 120 per hour. If he works x hours, then for what value of x does the first scheme give better wages?
Solution:
Let the number of hours to complete the job = x
Wages for the first scheme = Rs. 500 + Rs. 70 per hour = 500 + 70x
Wages for the second scheme = Rs. 120 per hour = 120x
Let us find the value of x for which the first scheme gives better wages.
500 + 70x > 120x
500 > 120x – 70x
500 > 50x
\(\frac{500}{50}\) > x
x < 10
∴ The value of x so that the first scheme gives better wages is = 1, 2, 3, 4, 5, 6, 7, 8, 9
Question 10.
A and B are working on similar jobs but their annual salaries differ by more than Rs 6000. If B earns Rs. 27000 per month, then what are the possibilities of A’s salary per month?
Solution:
Let A’s salary be x, B’s salary is Rs. 27,000
Given their difference in salary is more than Rs. 6,000
Assume A’s salary is more than B’s salary.
∴ x – 27,000 > 6000
∴ x > 6000 + 27000
x > 33000
Assume B’s salary is more than A’s salary.
∴ 27,000 – x > 6000
∴ 27000 – 6000 > x
x < 21000
The possibilities of A’s salary are greater than Rs. 33,000 or less than Rs. 21,000.
Samacheer Kalvi 11th Maths Solutions Chapter 2 Basic Algebra Ex 2.3 Additional Questions
Question 1.
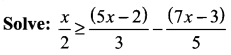
Solution:

Multiplying both sides by 30, we get 15x ≥ 2(4x – 1) ⇒ 15x ≥ 8x – 2⇒ 15x – 8x ≥ -2

Question 2.
Ravi obtained 70 and 75 marks in the first two unit tests. Find the minimum marks he should get in the third test to have an average of at least 60 marks.
Solution:
Let x be the marks obtained by Ravi in the third test.
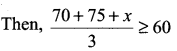
⇒ 145 + x ≥ 180 ⇒ x >180 – 145
⇒ x ≥ 35
Thus, Ravi must obtain a minimum of 35 marks to get an average of at least 60 marks.
Note. A minimum of 35 marks.
⇒ Marks greater than or equal to 35.
![]()
Question 3.
To receive Grade ‘A’ in a course, one must obtain an average of 90 marks or more in five examinations (each of 100 marks). If Sunita’s marks in the first four examinations are 87, 92, 94, and 95, find minimum marks that Sunita must obtain in the fifth examination to get Grade ‘A’ in the course.
Solution:
Let x be the marks obtained by Sunita in the fifth examination. Then,
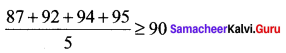
⇒ 368 + x ≥ 450
⇒ x ≥ 450 – 368
⇒ x ≥ 82
Thus, Sunita must obtain marks greater than or equal to 82,
i.e., a minimum of 82 marks.
Question 4.
Find the pairs of consecutive odd positive integers both of which are smaller than 10 such that their sum is more than 11.
Solution:
Let x be the smaller of the two consecutive odd positive integers, then the other is x + 2.
According to the given conditions.
x < 10, x + 2 < 10 and x + (x + 2) > 11
⇒ x < 10, x < 8
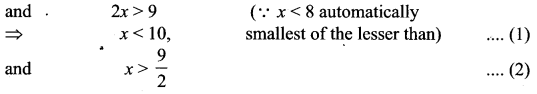
From (1) and (2), we get 9
\(\frac{9}{2}\) < x < 8
Also, x is an odd positive integer. x can take values 5 and 7.
So, the required possible pairs will be (x, x + 2) = (5, 7), (7, 9)
![]()
Question 5.
Find all pairs of consecutive even positive integers, both of which are larger than 5 such that their sum is less than 23.
Solution:
Let x be the smaller of the two consecutive even positive integers, then the other is x + 2. According to the given conditions.
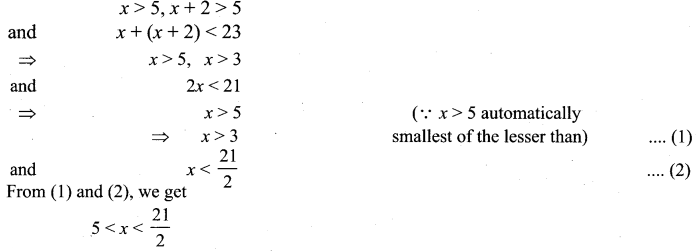
Also, x is an even positive integer.
x can take the values 6, 8, and 10.
So, the required possible pairs will be (x, x + 2) = (6, 8), (8, 10), (10, 12)
Question 6.
Forensic Scientists use h = 61.4 + 2.3F to predict the height h in centimetres for a female whose thigh bone (femur) measures F cm. If the height of the female lies between 160 to 170 cm find the range of values for the length of the thigh bone?
Solution:
Given h = 61.4 + 2.3 F
Given h = 160
⇒ 160 = 61.4 + 2.3 F
⇒ 2.3 F = 160 – 61.4 = 98.6
![]()
Given h = 170 ⇒ 170 = 61.4 + 2.3 F
⇒ 170 – 61.4 = 2.3 F
2.3F = 108.6

So the ranges of values are 42.87 < x < 47.23